Video Transcript
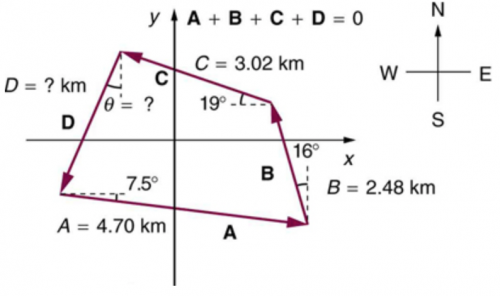
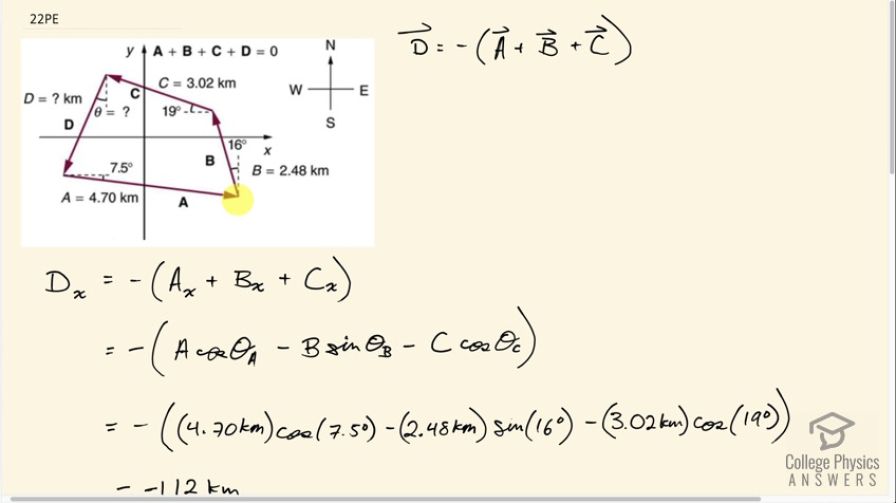
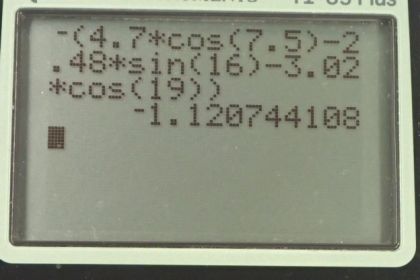
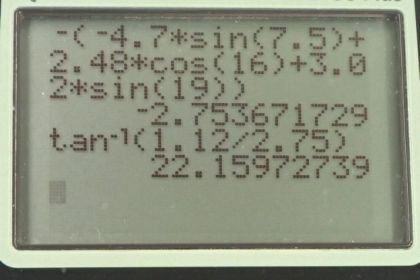
This is College Physics Answers with Shaun Dychko. A farmer is going to enclose their field with these four sides and they start here then they move along vector A and then along B, and then along C. Noting the direction and length for each of those sides, then the question is figure out what this fourth side labeled letter D should be. And with an angle here with respect to the south, to the west of south, and this length that we’ll have to figure out. So if we were to add these three vectors together the resultant would be from the starting point to the ending point. And this resultant would have the same length and angle as the vector D that we want to find pointing this way, just in the opposite direction. So what we're going to do is add vectors A, B, and C together and we have techniques for doing that. We're going to use the adding components technique in this video. And then once we get that resultant, we’ll take the opposite direction of it to get our answer. And the opposite direction we can find by putting a negative sign in front of it. So vector D then is going to be the opposite which is to say a negative of the sum of vectors A, B, and C. So, the component in the x-direction for this vector D is going to be the opposite of adding all the components of vectors A, B, and C together. So the x-component for vector A is going to be the adjacent leg of this triangle. So this horizontal part here is Ax component and that's the adjacent leg of this triangle going all the way to the dotted line there, down here and along vector A. A, B and the hypotenuse of this triangle in yellow. And this is being the adjacent leg, means we’ll have to use cosine of this angle theta A which is 7.5 degrees, multiplied by the hypotenuse which is the length of A, 4.7 kilometers. And this is to the right and so that makes it positive. And vector B is somewhat to the left. Let's get rid of all this here. Vector B is somewhat to the left and so its component is going to be negative, it’s this little bit here Bx and that's the opposite leg of this triangle. And so we’ll use the sine of this angle here theta B, which is 16 degrees and multiply by the hypotenuse. I didn't quite draw that triangle very well, it goes all the way to the end of vector B. That’s better, and this is the y-component By. And then here is the x-component Bx. And this, this is the triangle we're concerned with here. So, this is the opposite leg of this triangle and we’ll use sine then, multiply it by the hypotenuse to find Bx. And we have this minus sign here because Bx is pointing to the left in the negative direction. And then vector C has an x-component which goes like this and then a y-component upwards and then I'll highlight that triangle. And we can see that we're given this angle 19 with respect to the horizontal and so we’ll use cosine of that angle multiplied by this hypotenuse to find the x-component for vector C. And that's pointing to the left and so we have a minus there. So let me plug-in all the numbers that were given on this picture: So 4.7 kilometers for vector A length, multiply by a cosine of 7.5 degrees, minus 2.48 kilometers for vector B’s length, times sine of 16 degrees, and then minus 3.02 kilometers for vector C’s length, times by cosine of 19 degrees. And all this together would give us the sum of the x-components for A, B, and C. And we want the opposite sign of that sum to get this factor D x-component. And so we have negative 1.12 kilometers and so we've discovered that this vector D has an x-component along here Dx, and here's Dy by the way. We can see that Dx is pointing to the left and so we expect it to be negative and sure enough it is, that's good. And it's an amount of 1.12 kilometers to the left. Okay the y-component for vector D is the same idea but we're now finding the y-components of each of the triangles here. Redraw the vector A triangle all the way to here and then a little bit down. Here’s Ay and here’sAx. So Ay we can see is a bit negative and it's the opposite leg and so we can use sine of 7.5 degrees, times the length of A. So we have a minus here because it's directed downwards a bit, so negative A, times sine theta A, plus B, times cos theta B. This By is the adjacent leg of this triangle and so we're using cosine of 16, times the length of B to get B y-component. And that's pointed upward so it's a positive and likewise vectors C is, its y-component is going upwards. Here’s the y-component for C. And it's the opposite legs so we’ll use sine of this angle, times the length of C. And then we plug-in numbers, and that gives negative 2.75 kilometers is the y-component for D. Now the direction for this vector D is going to be the inverse tangent of the opposite which is Dx, divided by the adjacent which is Dy. And so we have inverse tangent of 1.12 kilometers, divided by 2.75 kilometers. And we can take the positives here because we already know what this direction is for this angle. It's this little bit in here and so we've already accounted for direction, so we don't need to put in our negative signs here inside of this inverse tangent to function. So we get 22.2 degrees to the west of south and then the length of vector D then, is the square root of its x-component squared, plus its y-component squared; so the square root of negative 1.12 kilometers squared, plus negative 2.75 kilometers squared, and we get 2.97 kilometers So our full answer than for vector D then is 2.97 kilometers, 22.2 degrees west of south.