Question
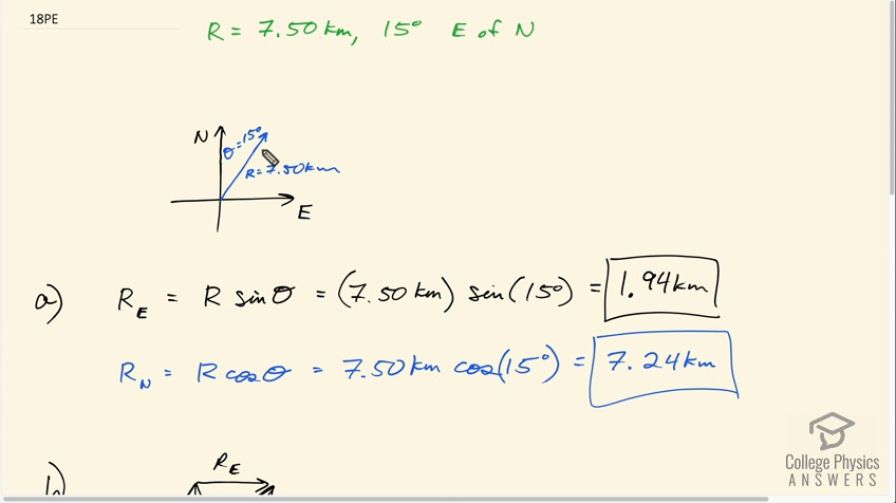
You drive 7.50 km in a straight line in a direction east of north. (a) Find the distances you would have to drive straight east and then straight north to arrive at the same point. (This determination is equivalent to find the components of the displacement along the east and north directions.) (b) Show that you still arrive at the same point if the east and north legs are reversed in order.
Final Answer
- ,
- Please see the solution video
Solution video
OpenStax College Physics, Chapter 3, Problem 18 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
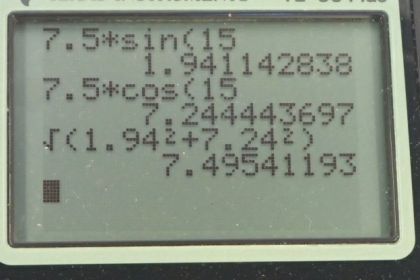
Video Transcript
This is College Physics Answers with Shaun Dychko. Imagine that we drive 7.5 kilometers, 15 degrees east of North. That's what's shown in this picture here. This angle is towards the east compared to north 15 degrees. And the length of this vector is seven and a half kilometers. The question to part A is what component of this vector is to the east and what component of it is north. That's another way of saying driving directly east and then directly north, which distances for those two directions would make you arrive at the same final place. We'll figure out the east of the component for this resultant vector then, and will say that it's R times sine of theta because this is the opposite leg of this triangle. We need sine of this angle multiplied by the hypotenuse of this right triangle to figure out the length of the opposite side. It's seven and a half kilometers time sine 15, which is 1.94 kilometers. Then the northerly component, which is the adjacent leg of that right triangle is going to be the hypotenuse R multiplied by cosine of theta. It's seven and a half times cos 15, which is 7.24 kilometers. Then in Part B, we're asked to verify that if you travel north and then east, will you arrive at the same position as you would have had you driven east and then north? Well, certainly, this, R prime, its length is going to be the square root of the northern component squared plus the east of the component squared. It's the square root of 7.24 kilometers squared plus 1.94 kilometers squared, which is 7.50 kilometers. Then the angle is going to be the inverse tangent of the opposite divided by the adjacent. It's the inverse tangent of 1.94 kilometers divided by 7.24 kilometers which is 15.0 degrees. That is towards the east compared to north. We have R prime then, this resultant that we get from driving first north and then east is seven and a half kilometers, 15 degrees east of North, which is the same as the resultant that we started with in part A. Yes, we verified that the order in which you drive along these components does not matter.