Question
Unreasonable Results Suppose you wish to shoot supplies straight up to astronauts in an orbit 36,000 km above the surface of the Earth. (a) At what velocity must the supplies be launched? (b) What is unreasonable about this velocity? (c) Is there a problem with the relative velocity between the supplies and the astronauts when the supplies reach their maximum height? (d) Is the premise unreasonable or is the available equation inapplicable? Explain your answer.
Final Answer
- or, Mach 78
- Mach 78 is not attainable on Earth.
- The astronaut will be orbiting, so the timing will need to be impossibly precise.
- The supplies should be made to orbit also to give a larger window of opportunity to intersect the path of the astronaut. Also, the assumption that "g" is constant over 36000 km above the Earth's surface is false.
Solution video
OpenStax College Physics, Chapter 3, Problem 68 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
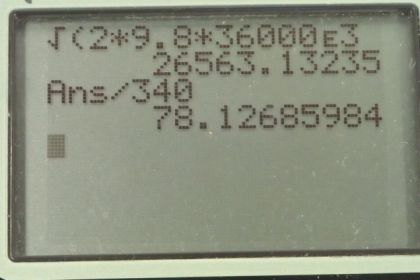
Calculator Screenshots
Video Transcript
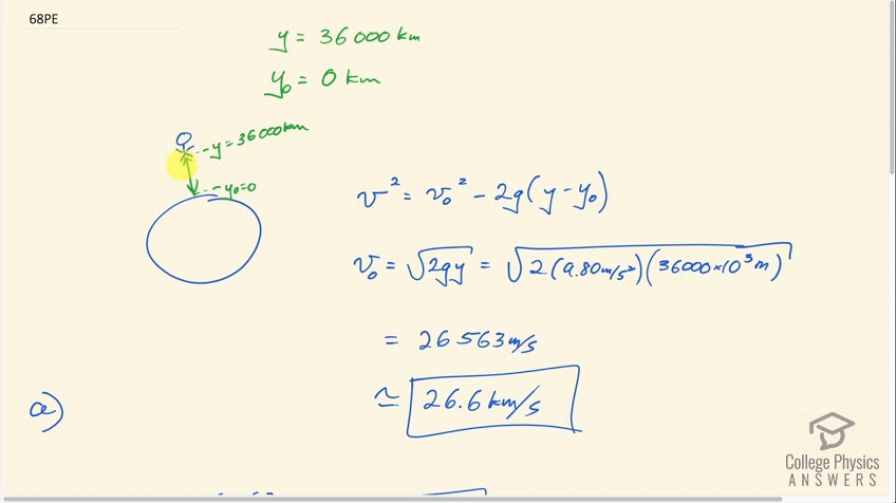
This is College Physics Answers with Shaun Dychko. We’re going to calculate the hypothetical speed that a parcel of supplies should be shot up from the surface of the Earth to reach an astronaut who is 36000 kilometers above the Earth’s surface. So the final height will be 36000 and the initial height, y naught would be zero. Then we have this equation here, which is number 75 in chapter two. Which says the final speed squared equals the initial speed squared, minus two times acceleration due to gravity. Times the change in position. So the final velocity will be zero, when the package gets to its maximum height and the initial position is zero. And then we can solve for v naught squared by adding two g y to both sides. And then we take the square root of both sides to solve for v naught. So v naught is the square root of two g y; So that’s two times 9.8 meters per second squared, times 36000, times ten to the three meters. And I converted the kilometers into meters here in order to match with the units in our acceleration due to gravity here. And we take the square root of that product and we get 26563 meters per second, which is 26.6 kilometers per second. Now to put that speed into perspective, we can turn it into a mach number and we can divided by the speed of sound 340 meters per second. And this would be mach 78. And mach 78 is not attainable on Earth. Fighter jets can go maybe mach eight or so, but not mach 78. And now another problem here is that the astronaut will be orbiting. Because it can’t just stay in one spot above the Earth otherwise they would fall straight towards the Earth. So since they are moving to the side, they have some high speed in order to maintain this orbit. And the supplies since we're going straight up, they have no horizontal component to their velocity. And at this moment when they're at the astronauts height, the supplies will have zero velocity. And this makes a very small window of opportunity for the astronaut to capture the supplies. Because right after this moment in time pictured here, the supplies will begin to fall back to Earth. And if the timing isn’t absolutely precise, the supplies won’t be here at the same time as the astronaut is. And so the package should be made to orbit just like the astronaut is, to give a bigger window of opportunity to intersect with the path of the astronaut. And the assumption that g, acceleration due to gravity is a constant over 36000 kilometers is false. g will actually be less than 9.8 at this large distance above the surface of the Earth.