OpenStax College Physics for AP® Courses, Chapter 21, Problem 60 (Problems & Exercises)

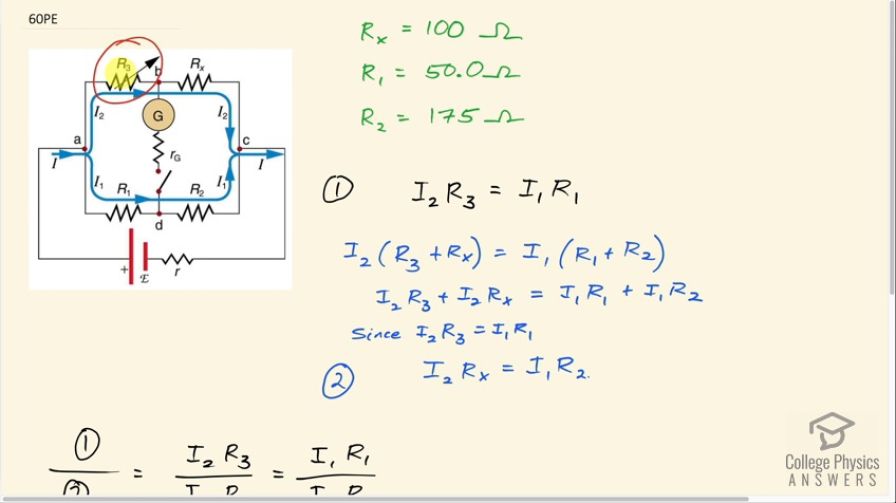
This is College Physics Answers with Shaun Dychko. Given this Wheatstone bridge circuit, we want to figure out what resistance should resistor three be given that we know this R x is 100 ohms, R 1 is 50.0 ohms and R 2 is 175 ohms? So this resistance R 3 is adjusted such that the current through this galvanometer is zero— the switch is closed by the way— and when that's true when there's no current through here, it means the potential at b is the same as the potential at point d in which case the potential difference between ab is the same as that between a and d or you could put it in these terms by saying I 2 multiplied by R 3 is the same as I 1 multiplied by R 1 that is the voltage across this resistor R 3 is the same as the voltage across resistor R 1. Okay! And then you can also say that well because this top entire branch is in parallel with this bottom entire branch that current I 2 through the top branch multiplied by its total resistance of the top branch R 3 plus R x equals the voltage across the bottom branch which is I 1 multiplied by its total resistance R 1 plus R 2 and then you can distribute the currents into the brackets and you have I 2R 3 plus I 2R x equals I 1R 1 plus I 1R 2. Now from this equation here, we know that I 2R 3 is the same as I 1R 1 so this voltage is the same as this voltage and so this number is the same as this number so you can subtract it from both sides and it makes zero leaving us with I 2R x equals I 1R 2. Then... by the way we are trying to solve for R 3 here so what we will do is divide these two equations— divide equation one by equation two— and we will have the left side is I 1R 3 divided by I 2R x and right hand side will have I 1R 1 divided by I 1R 2 and the I 2's cancel and the I 1's cancel and we can multiply both sides by R x and then we get an equation for R 3: it's R x times R 1 divided by R 2. So that's 100 ohms times 50.0 ohms divided by 175 ohms which is 28.6 ohms.