Question
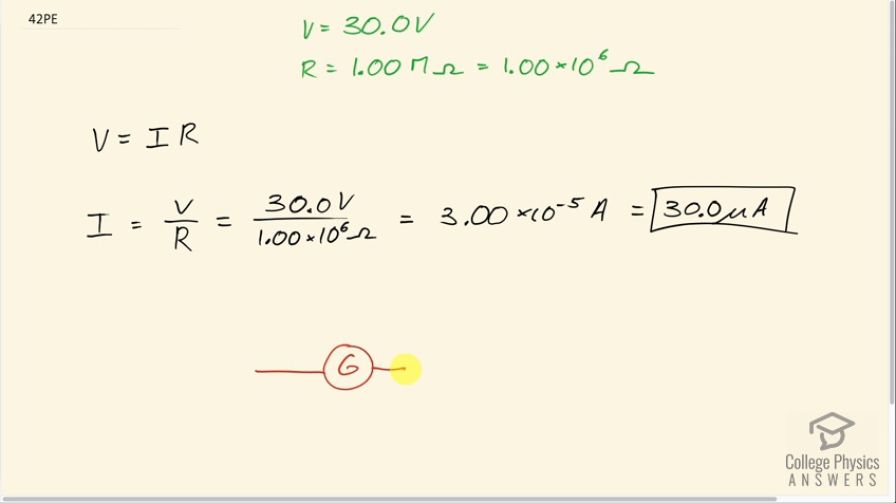
What is the sensitivity of the galvanometer (that is, what current gives a full-scale deflection) inside a voltmeter that has a 1.00-M Ω resistance on its 30.0-V scale?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 42 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A voltmeter contains a galvanometer— this would be a schematic of a voltmeter; there's a galvanometer and there's its resistance inside— and the resistance is kept as large as possible to avoid changing the voltage that it's trying to read in this other circuit. So the question here is what is the sensitivity of the galvanometer or in other words, what current causes a full scale deflection on the galvanometer such that we have a 30.0 volt reading and the resistance is 1.00 megaohm, which is 1.00 times 10 to the 6 ohms? So we have Ohm's law here it says that the voltage across these terminals equals the current through the galvanometer times the resistance and we can solve for I then and we divide both sides by the resistance and this solves for I equal V over R. So that's 30.0 volts at full scale deflection divided by 1.00 megaohm and that's 30.0 microamps. So this is the current that causes the needle in the galvanometer to go you know, full scale so it kinda looks like a little gas gauge inside there and then you will have different numbers— you know, 0 up to 30— and when the galvanometer is reading 30, the current through it is 30.0 microamps and so the sensitivity is 30.0 microamps.
