Question
The hot resistance of a flashlight bulb is , and it is run by a 1.58-V alkaline cell having a internal resistance. (a) What current flows? (b) Calculate the power supplied to the bulb using . (c) Is this power the same as calculated using ?
Final Answer
- Yes, gives the same answer.
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 21 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
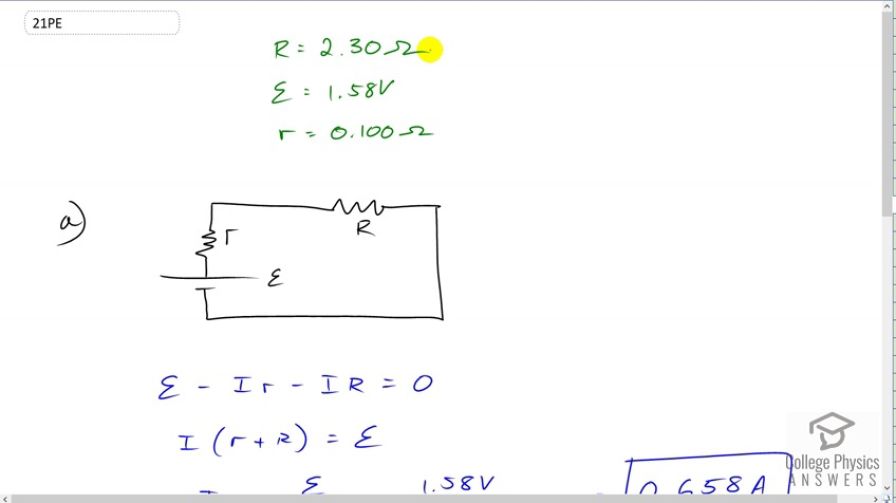
This is College Physics Answers with Shaun Dychko. The hot resistance of the flash bulb is 2.30 ohms. To say hot resistance just means it's the resistance of the bulb when there is current going through it and it's heated up because its resistance will change with temperature. So we're told this is the resistance when it's at its running temperature, it's hot, its high temperature. The EMF of the battery is 1.58 volts and it has an internal resistance of 0.1 ohms. The question is what current is going through the battery in this case. So using the Loop Rule, we say that the positive EMF -- we have current going this way by the way -- minus the potential drop across the internal resistance which current times small r, then minus the current times capital R, all of this equals zero. So we can add I little r and I capital R to both sides and then factor out this common factor I and switch the sides around. We get I times r plus R equals EMF. So we divide by little r plus capitalR, this is internal resistance plus flash bulb resistance, and we get I equals EMF divided by total resistance. So that's 1.58 volts divided by 0.10 plus 2.3 ohms which gives 0.658 amps. Now part B asks the power supplied by the bulb -- power supplied to the bulb I should say -- so the power supplied to the bulb is going to be the current through it squared, multiplied by the resistance of the bulb. So we just calculated the current here and we square that and multiply by 2.3 ohms, resistance of the bulb, and we get 0.997 watts. Then we're asked to calculate that value again, the power supplied to the bulb using the V squared over R formula. So the voltage across the bulb is the terminal voltage and that is going to be EMF minus the current times the internal resistance. We square that and divide by R. So we have 1.58 volts minus 0.65833 amps times 0.1 ohms and square that difference and divide by 2.3 ohms and we get 0.997 watts which is indeed the same answer, just using a different formula.
