Question
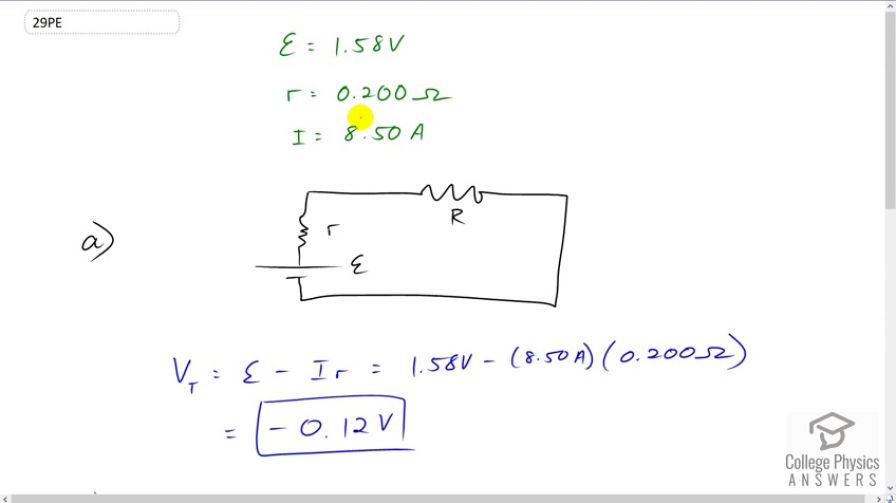
A 1.58-V alkaline cell with a internal resistance is
supplying 8.50 A to a load. (a) What is its terminal voltage? (b) What is the value of the load resistance? (c) What is unreasonable about these results? (d) Which assumptions are unreasonable or inconsistent?
Final Answer
- Terminal voltage and resistance should not be negative.
- The current is too high. The maximum possible current is
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 29 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a circuit consisting of an alkaline cell with an EMF of 1.58 volts and an internal resistance of 0.2 ohms. There is a load resistance as well which we're not given but we're told what the current is through the circuit. It's eight and a half amps. So the question is in part A, what is the terminal voltage of this battery? Well it's going to be the EMF minus the current through the battery times its internal resistance. So that's 1.58 volts minus eight and a half amps times 0.2 ohms which is negative 0.12 volts. Now that's a bit of a weird answer but we'll get to that in a second. Now the voltage across the load is the same as the terminal voltage because the load is connected to here and to here by wires that have no resistance. So the voltage across the load is the current times the resistance of the load and we divide both sides by I to solve for R. So capital R is terminal voltage over current which is negative 0.12 volts divided by eight and a half amps which is negative 0.0141 ohms and that is impossible. Terminal voltage and resistance both should be positive. They should not be negative. The reason for this problem is that the current we're given of eight and a half amps is higher than what's possible for this battery because the current is going to be the EMF of the battery divided by the total resistance of the circuit which is the internal resistance plus the load resistance. We can solve for what is the maximum possible current by saying let's suppose there's a short circuit and the load resistance is zero, in which case the maximum current would be the EMF divided by the internal resistance which is 1.58 volts divided by 0.2, which is 7.9 amps. Any number than 7.9 amps is impossible and they've given us 8.5 in this question.
