Question
There is relatively little empty space between atoms in solids and liquids, so that the average density of an atom is about the same as matter on a macroscopic scale—approximately . The nucleus of an atom has a radius about that of the atom and contains nearly all the mass of the entire atom. (a) What is the approximate density of a nucleus? (b) One remnant of a supernova, called a neutron star, can have the density of a nucleus. What would be the radius of a neutron star with a mass 10 times that of our Sun (the radius of the Sun is )?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
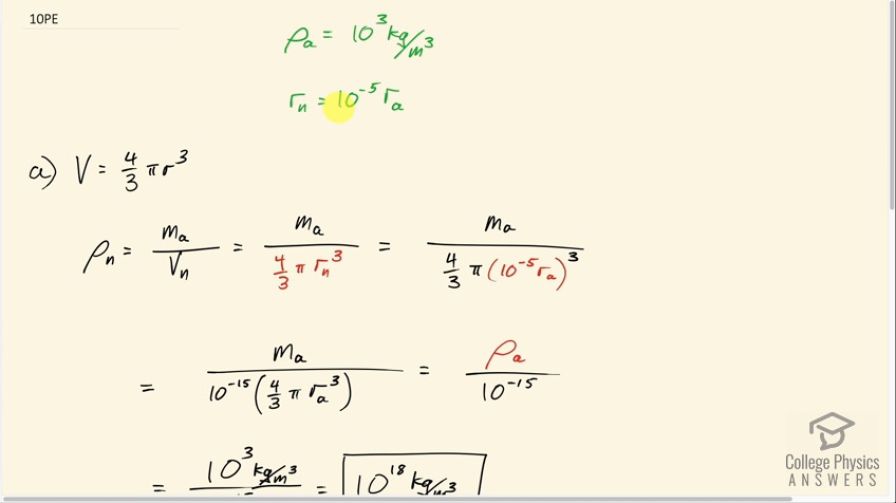
This is College Physics Answers with Shaun Dychko. In this question, we want to find out what the density of a nucleus is and we are told that the radius of a nucleus is about 10 to the minus 5 multiplied by the radius of the whole atom and that pretty much all of the mass of the atom is in the nucleus and so when we are calculating the density of a nucleus, we can take the mass of the whole atom divided by the volume of the nucleus. And we'll assume that the nucleus is a sphere in which case its volume is four-thirds π times its radius cubed so I make that substitution here. And then replacing r n, which is the radius of the nucleus, with 10 to the minus 5 times the radius of the atom and take this 10 to the minus 5 out of the bracket, cube it and it becomes 10 to the minus 15 and we have this whole thing is the density of an atom and we are told what that density is here. So we have the density of a nucleus is about the density of an atom— 10 to the 3 kilograms per cubic meter— divided by 10 to the minus 15 which is 10 to the 18 kilograms per cubic meter. In part (b), it says what would the radius be of a neutron star assuming it has the same density as a nucleus and it has a mass 10 times that of the Sun? So its mass then is gonna be 10 times 1.99 times 10 to the 30 kilograms so that's 1.99 times 10 to the 31 kilograms and its density is mass divided by its volume— volume being four-thirds πr cubed assuming its a sphere—and we can multiply top and bottom by 3 here... the 3's cancel and we are left with 3M n over 4πr cubed and then we'll solve this for r by multiplying both sides by r cubed over ρ and then take the cube root of both sides. So we have r is a cube root of 3 times mass of the neutron star divided by 4π times its density and its density we are told is the same as that of a nucleus— 10 to the 18 kilograms per cubic meter. So we plug in numbers, raise it to the power of one-third, which is the same as cube rooting, and we get 2 times 10 to the 4 meters would be the neutron star's radius.
