Question
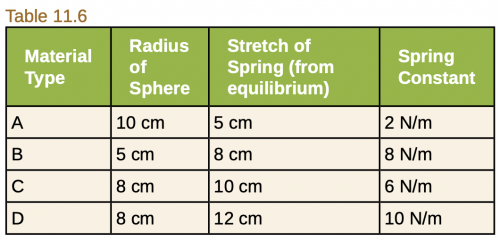
Four spheres are hung from a variety of different springs. Table 11.6 below describes the characteristics of both the spheres and the springs from which they are hung. Rank the densities of the objects listed below, from greatest to least. Show work supporting your ranking.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 11, Problem 4 (Test Prep for AP® Courses)

vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
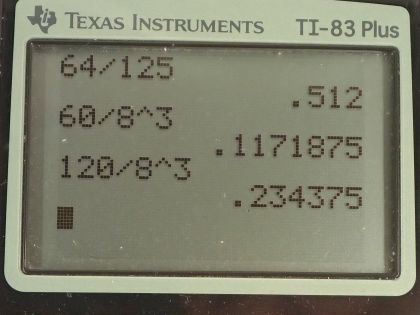
This is College Physics Answers with Shaun Dychko. Four spheres of different radii are going to be hanging from a spring and we have different spring constants and different amounts of stretching of the spring and we have to figure out what the ranking is for density among these different materials. So when the mass is hanging from the spring, it stretches some distance Δx from equilibrium at which point, the spring force upwards equals gravity downwards and that is the spring constant times the amount of stretching Δx equals the mass of this thing times gravitational field strength and we can solve for m by dividing both sides by g and this is going to be the numerator in our density formula so density is mass divided by volume. The volume is four-thirds π times the radius of the sphere cubed and so we have kΔx over g substituted in place of m and then we are dividing by volume and so I am going to multiply by its reciprocal because dividing a fraction by a fraction gets messy so we are multiplying by 3 over 4πr cubed in which case we have density is 3kΔx over 4πgr cubed all of this... this 3 over 4πg can be summarized in a single factor we'll call Z and this Z will also take care of unit conversions because we have some non-mks units here normally, we would have meters, kilograms and seconds in our units but here we have some centimeters instead of meters but that's not gonna matter because we are not trying to find the density in kilograms per cubic meter here, we are just trying to figure out what the ranking of densities are. So we'll have this factor Z include all of the things that would be needed hypothetically to convert to units of kilograms per cubic meter. So for the density of sphere A, it's gonna be Z times the 2 newtons per meter—spring constant— substituted in place of k times 5 centimeters of stretching divided by 10 cube— that's the radius 10 centimeters here— I didn't write in units again because our units are a bit weird and we don't care about the units in this context. So we end up with 10 over 10 cubed and that is 0.01 Z— that's the density for ball A. For sphere B, we have Z times 8 centimeters of stretch times 8 newtons per meter spring constant divided by a radius of 5 cubed and that is 0.512 times Z; density for C is Z times 6 times 10 divided by 8 cubed that is 0.117 Z and density for ball D is 10 times 12 divided by 8 cubed and that's 0.234 Z and so our ranking then is material B is the highest density at 0.512 Z and then followed by the material D, which has the density of the next biggest factor times Z 0.234 times Z and then this material C is next and then the lowest density material is A.