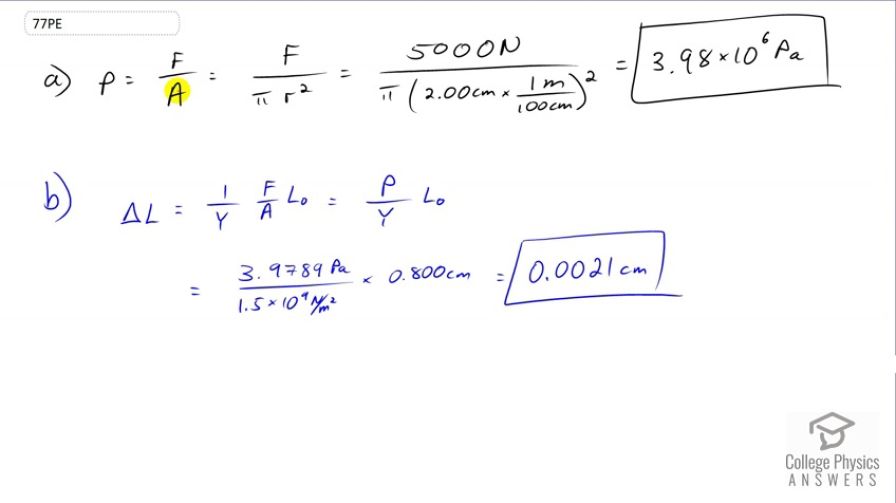
During heavy lifting, a disk between spinal vertebrae is subjected to a 5000-N compressional force. (a) What pressure is created, assuming that the disk has a uniform circular cross section 2.00 cm in radius? (b) What deformation is produced if the disk is 0.800 cm thick and has a Young's modulus of $1.5\times 10^9{\text{ N/m}}^2$?

Video Transcript

This is College Physics Answers with Shaun Dychko. So the question here is what pressure is being exerted on the disks in the back? So we divide the force by the area of a disk. So that's 5000 newtons divided by pi times the radius squared. The radius is two centimeters converted into meters by multiplying by one meter for every 100 centimeters. We square that and we get 3.98 times ten to the six Pascals. Now the amount of compression of the disk will be one over the Young's Modulus and this formula is from chapter 5 by the way, we multiply by the pressure, force per area and then multiply by the initial length. So this is basically pressure over Young's Modulus times l naught. So that's 3.9789 Pascals divided by 1.5 times ten to the nine newtons per square meter which is the Young's Modulus for a disk in the back. Multiply that by 0.8 centimeters and you get 0.0021 centimeters of compression which is very little.