Question
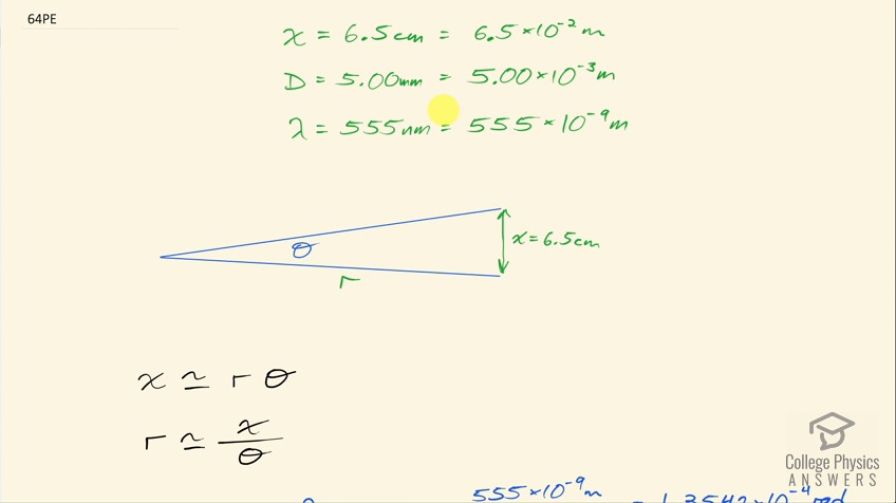
You are told not to shoot until you see the whites of their eyes. If the eyes are separated by 6.5 cm and the diameter of your pupil is 5.0 mm, at what distance can you resolve the two eyes using light of wavelength 555 nm?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 64 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A hunter has been told that they should not shoot their prey unless they can see the whites of the eyes of the deer or the moose or whatever it is they are hunting so they need to be able to distinguish between the eyes of this creature and the eyes are separated by 6.5 centimeters and we can figure out what the minimum angle that's possible here based on the diameter of the person's pupil and the average wavelength of light that they are seeing with and figure out what this distance r should be. So this r will be the maximum distance the animal could be away. Okay! So this distance x here is pretty much the same as the arc length between these two points here; we imagine that there's a big circle and this angle Θ is the ratio of the arc length, which would be s divided by the radius r. Now for a very small angle, this arc length s would be pretty much the same as a straight line distance between the two eyes, which is labeled x. So we'll say x is approximately the same as r times Θ because you know s, if we were to multiply both sides by r here, s would be r times Θ and we are saying s is approximately the same as x. Alright! So this distance x is approximately the distance to the animal times this minimum angle based on the wavelength of light and the diameter of the person's eye. We can solve for r by dividing both sides by Θ and we have r is x over Θ so we need to figure out this Θ and this is from The Rayleigh Criterion. It's 1.22 times the wavelength of light divided by the diameter of the eye so that's 1.22 times 555 times 10 to the minus 9 meters divided by 5.00 times 10 to the minus 3 meters and that gives 1.3542 times 10 to the minus 4 radians and so we take the separation between the eyes of the creature divided by that angle in radians and the distance then is 480 meters. So the animal has to be at least this close before it's worthwhile shooting them; if the animal's farther away from that I guess the thinking goes that it's too risky to miss or hit the wrong spot so it has to be at least this close before shooting it.
