Question
(a) What is the smallest separation between two slits that will produce a second-order maximum for any visible light? (b) For all visible light?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 16 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
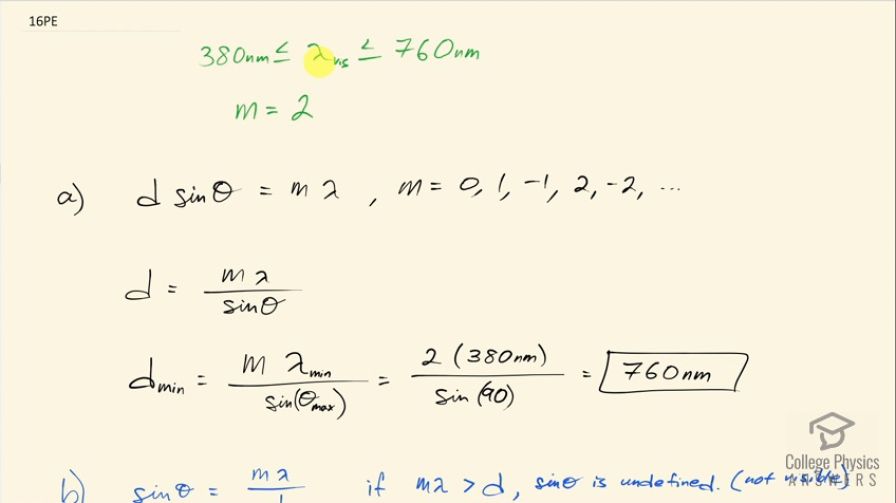
This is College Physics Answers with Shaun Dychko. What is the smallest separation between two slits that will produce a second order maximum for any visible light? So second order maximum means m equals 2 and here is the possible spectrum for visible light: it goes from 380 nanometers at its shortest to a maximum wavelength of 760 nanometers. This is the formula for constructive interference or maxima in the double slit interference pattern. So we have d is the separation between the slits times sin of the angle to the maximum equals the order of the maximum times the wavelength of the light and we can solve this for d by dividing both sides by sin Θ and we want to know what is the minimum distance between the slits? And so that means we want to minimize the numerator and maximize the denominator so the sin of Θ has a maximum when Θ is at its maximum of 90 degrees or another way to say it is that the range of sin Θ— whatever Θ is— the range is at a maximum of 1 the amplitude of sin Θ is 1. Okay! So the denominator's 1 and the numerator the minimum possible wavelength is 380 nanometers so we have 2 times 380 nanometers divided by 1 and that's 760 nanometers. Part (b) is saying get the minimum separation between slits again but this time make sure that every single wavelength is possible to see. Now I have rearranged this maximum formula—the position of the maxima— I have rearranged it to solve for sin Θ by dividing both sides by d and we have sin Θ is mλ over d and I am doing this to explain that there are some wavelengths that willl not be visible given this minimum separation between slits So sin Θ is mλ over d now the maximum possible value for sin Θ is 1 and that means this numerator cannot be more than the denominator because if the top of the fraction is more than the bottom that will make the fraction greater than 1 which is possible but not possible if you want it to equal sin Θ and the physical interpretation of having this number greater than 1 is that the maximum will not be visible. Okay! So if mλ is more than d if the numerator is more than the denominator then sin Θ is undefined and not visible. Okay! So if you have a wavelength with this separation more than 380 then that number times 2 will exceed 760 and so in that case you would not be able to see that maximum. So all this to say that you want to have the maximum wavelength that's needed in our spectrum multiplied by the order divided by the maximum value of sin Θ. So the maximum wavelength is 760 nanometers at the red end of the spectrum times that by 2 divided by 1 and that's 1520 nanometers is the minimum separation needed in order to have a first order maximum for every wavelength of visible light.