Question
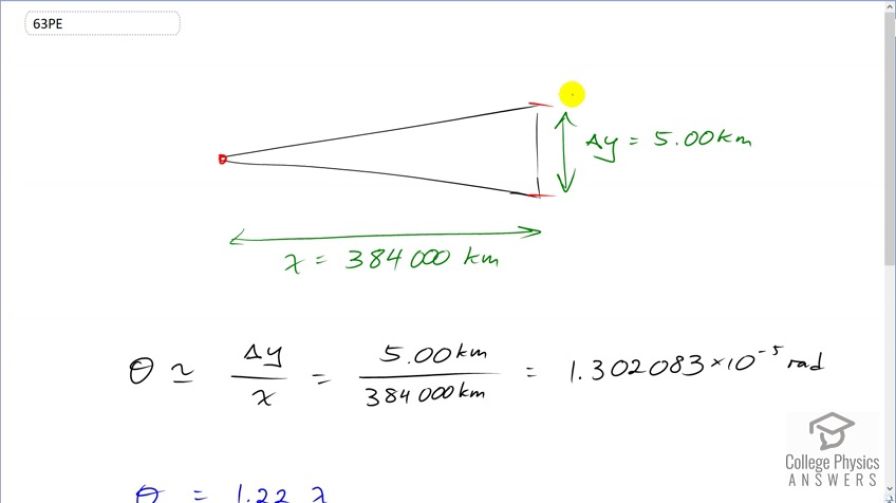
What is the minimum diameter mirror on a telescope that would allow you to see details as small as 5.00 km on the Moon some 384,000 km away? Assume an average wavelength of 550 nm for the light received.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 63 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. With the telescope on Earth here, we want to be able to distinguish between two objects that are separated by five kilometers over here on the moon and the moon is 384,000 kilometers away. And so what is this angle here. And we're going to use the formula for the really criterion and knowing the angle and the wavelength which we're told is about 550 nanometres. Figure out what minimum diameter of telescope you need in order to be able to resolve those objects separated by five kilometers on the moon. So theta is approximately going to be this separation divided by x and we can take the use this approximation because the angle is really small. x is really large compared to delta y in other words. So five kilometers divided by 384,000 kilometers is 1.302083 times ten to the minus five radians. If you don't like this approximation you could instead turn this into two right triangles then take the inverse tangent of this which would be half of delta y divided by x and then multiply that by two and then you would get the same answer to this many significant figures. So we'll rearrange this really criterion formula by multiplying both sides by d over theta to solve for the diameter of the telescope that will need. So it’s 1.22 times the wavelength over the angle in radians. So it's 1.22 times 550 times ten to the minus nine meters wavelength divided by 1.30203 times ten to the minus five radians giving a diameter of 5.15 centimeters is the minimum needed in our telescope.
