Question
Suppose you have a lens system that is to be used primarily for 700-nm red light. What is the second thinnest coating of fluorite (magnesium fluoride) that would be non- reflective for this wavelength?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 76 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
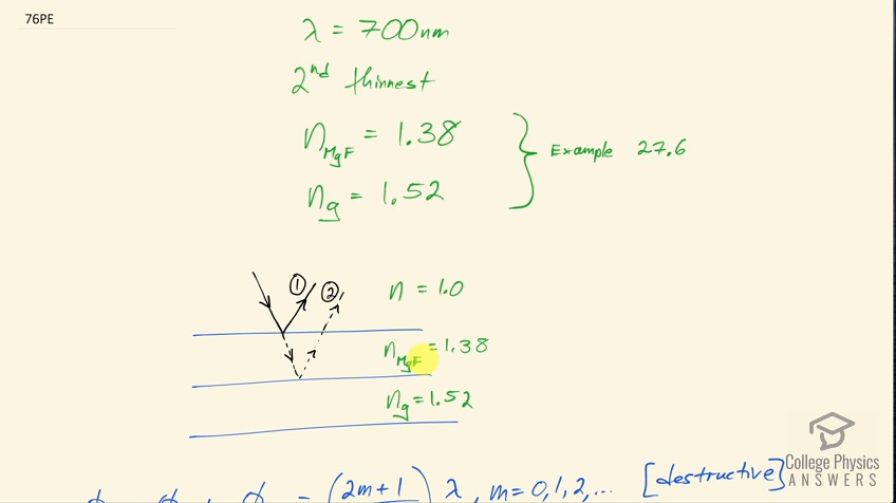
This is College Physics Answers with Shaun Dychko. In this question we want to find the second thinnest layer of magnesium fluoride or also known as fluorite which is an anti-reflective coating that we are going to put on top of glass to block the 700 nanometer wavelength from reflecting. So we want to create some destructive interference between this ray that is reflected from the surface of the coating versus this ray that's reflected from where the coating interfaces with the glass. So the index of refraction for fluorite is 1.38—I looked that up in example [27.6]— and the index of refraction of glass in the lens we'll take to be 1.52. Okay! So the total phase difference, which I label with this letter Φ is the phase shift of ray one plus the phase shift of ray two. When this total phase shift equals 2m plus 1 over 2 times the wavelength then we'll have destructive interference where this m can be any whole number— 0, 1, 2 and so on. So this fraction creates, you know, one-half λ, three halves λ, five halves λ and so on. Okay! So the phase shift of this first ray after reflection is half of a wavelength because this light is going from a low index of 1.0 in the air and the second medium has a higher index of refraction 1.38 so when the second medium at an interface is at a higher index of refraction, the reflection at that interface automatically has a one-half wavelength phase shift. And the phase shift of this second ray is due to two parts one is the same reflection effect here because it's going from low index of 1.38 to a medium of higher index 1.52 and so the reflection here also has a λ over 2 phase shift and in addition to that there is a greater path length traveled by ray two which also causes a phase shift. and that's going to be 2 times the thickness of this anti-reflective coating because it does two trips—one down and then once back up and that's why it's multiplied by 2— and the number of wavelengths that will be is dividing by the wavelength that exists in this material. So that's the number of wavelengths shifted and then we multiply that by the wavelength in air to get the phase shift in the air. Okay! So the wavelength in the anti-reflective coating is the wavelength in a vacuum or in air— they are pretty much the same— divided by the index of refraction of the coating and so we are dividing by this fraction and in which case, I will multiply by its reciprocal so I am flipping this over and multiplying 2t by n over λ λ's cancel and we are left with λ over 2 plus 2 times the anti-reflective coating thickness times the index of reflection of fluorite. So the total phase shift then is going to be λ over 2 from the first ray reflection plus λ over 2 plus 2tn and that has to equal 2m plus 1 over 2 times λ in order for destructive interference. So we can add these two terms together to get 2λ over 2 and then we are going to subtract 2λ over 2 from both sides and instead of calling this just λ, I left it as 2λ over 2 because now it has a common denominator with this term. And so we have 2tn equals 2m plus 1 over 2λ minus 2λ over 2 and then the λ gets factored out as well as the denominator 2 so we have λ over 2 times 2m plus 1 minus 2 and that works out to 2m minus 1. Okay! We are solving for t here so let's divide both sides by 2n and we get the thickness then is the wavelength divided by 4 times the index of refraction times 2m minus 1. So what should m be? Well we want the second thinnest so not the thinnest but the second thinnest. So you might think well 0 is the first permissible smallest value so we could try that but that would end up with a negative number here and you can't really have a negative thickness so zero is not the first thickness it's actually 1 is going to be the first thickness and so the second thickness is going to be the number two so we choose m equals 2. So the thickness then of the anti-reflective coating will be 700 nanometers divided by 4 times 1.38 times 2 times 2 minus 1, which is 380 nanometers.
