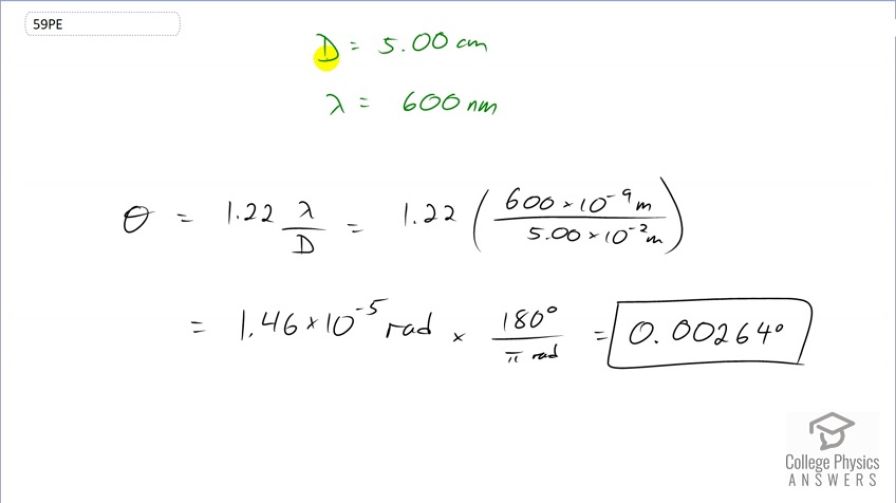
Question
Diffraction spreading for a flashlight is insignificant compared with other limitations in its optics, such as spherical aberrations in its mirror. To show this, calculate the minimum angular spreading of a flashlight beam that is originally 5.00 cm in diameter with an average wavelength of 600 nm.
Final Answer
Note: The working in the video is correct but the final answer in the video needs to be divided by . The final answer here and in the calculator screenshot are correct.
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 59 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In this question we're going to show that the diffraction spreading of a handheld flashlight is insignificant. So the size of the opening at the end of the flashlight the diameter of its aperture is five centimeters. And the average wavelength it's emitting is 600 nanometres. And so we use this really criterion formula to figure out the diffraction spreading the angle with which the beam is spreading outwards. So this is theta here and here is the flashlight. So we have 1.22 times the wavelength divided by the diameter of the aperture. So it's 1.22 times 600 times ten to the minus nine meters divided by five times ten to the minus two meters. Giving us this answer in radians which we multiply by 180 degrees for every Pie radians in order to get this answer in degrees 0.00264 degrees which is very small and you would never notice that without special instruments.
