Question
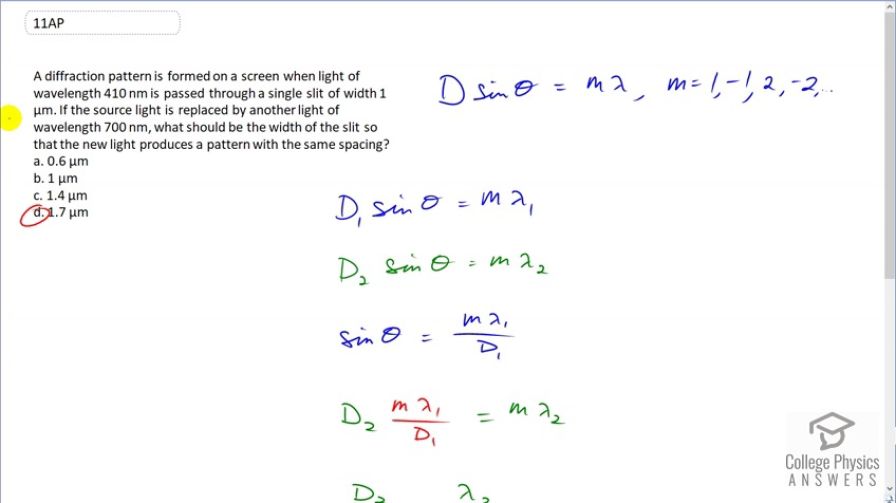
A diffraction pattern is formed on a screen when light of wavelength 410 nm is passed through a single slit of width 1 μm. If the source light is replaced by another light of wavelength 700 nm, what should be the width of the slit so that the new light produces a pattern with the same spacing?
Final Answer
(d)
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 11 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a diffraction pattern formed when 410 nanometer light is passed through a slit of with one micrometer. Now if this light is then replaced by a different one with wavelength 700 nanometres what should the width of this lid be adjusted to in order have the same spacing in the diffraction pattern? So the diffraction pattern is given by this formula. This tells us what the angle is to each of the dark fringes and it has the slit with capital D times sine of that angle equals m lambda where m is some integer one, negative one and so on and Lambda is the wavelength of light. So in the first case with the first slit with we’ll have D1 times sine theta equals m lambda 1 and in the second case we'll have D2 sine Theta and I intentionally did not rate a subscript 2 there because this angle is meant to be the same in the second case because we want to have the same pattern. So we have the same Sine Theta equals m lambda 2. Now from this first formula we can solve for sine theta and say that it m lambda 1 over D1 by dividing both sides by D1 here and then we'll use that to replace Sine Theta in the second expression. So I've written m lambda 1 over D1 in place of Sine Theta in this expression in the second scenario and so we have D2 over D1 equals lambda 2 over lambda 1 because we can multiply both sides by one over m lambda 1 and the m cancel and so D2 over D1 is lambda 2 over lambda 1 and then we'll multiply both sides by D 1 to solve for D2 so the diameter 2 such that we have the same pattern as we did with diameter or slit with one. I used the word diameter because of the D but it's not a really diameter it's the slit width so D2 is D1 times lambda 2 over lambda 1 so that's one micrometer initially times a new wavelength of 700 nanometres divided by the initial wavelength of 410 nanometres giving a new slit with of 1.71 micrometers to give the same diffraction pattern and so that's which one is that, that's answer D.
