Question
(a) Show that a 30,000-line-per-centimeter grating will not produce a maximum for visible light. (b) What is the longest wavelength for which it does produce a first-order maximum? (c) What is the greatest number of lines per centimeter a diffraction grating can have and produce a complete second- order spectrum for visible light?
Final Answer
- undefined
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 37 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
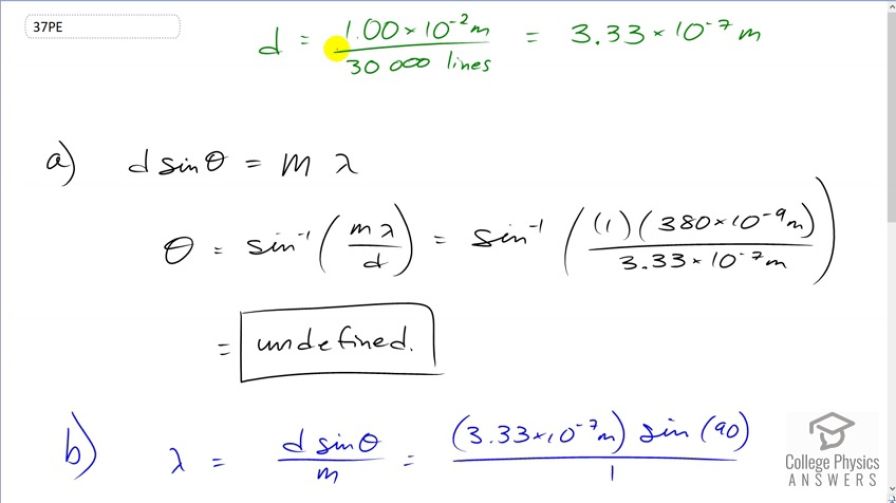
This is College Physics Answers with Shaun Dychko. We have a diffraction grating with 30,000 lines per centimeter. And let's find out the separation between each line. So we'll take the reciprocal of that and say one centimeter for every 30,000 lines and we'll write centimeters as one times ten to the minus two meters. This gives us 3.33 times ten to the minus seven meters between each line. So with that information we can answer a question a which says show that none of the wavelengths in the visible spectrum will appear in the diffraction pattern. So let's hypothetically say that it will appear and solve for the angle and we'll divide both sides by d and then take the inverse sine of both sides and we get that the angle to a maximum is the inverse sine of the order times a wavelength divided by d. So we want to minimize this number here in order to take the inverse sine of it. Because what we're trying to avoid is the possibility that this number is greater than one because the inverse sine is something greater than one is undefined because the sine graph is like this. And the range of the sine graph is between positive one and negative one, then when you take the inverse sine of a number you're finding out what is the x value corresponding to that particular sine value. But if this the sine is greater than one there is no x value that would correspond to it. So we're gonna take the inverse sine of one times the smallest wavelength we can put in here because we want to minimize this value and prevent it from going over one. So we put in 380 times ten to the minus nine meters. That's the wavelength of Violet, the minimum wavelength in the visible spectrum. And we divide by the separation between lines and the diffraction grating of 3.33 times ten to the minus seven meters. But we find that nevertheless this is undefined because this is a number greater than one. So there is no angle in the diffraction pattern for wavelengths that are in the visible spectrum. Then part b asks us for what wavelength would have a first order maximum for this diffraction grating. So we're solving this formula now for lambda by dividing both sides bym and we get lambda is separation between lines in the diffraction grating times sine over m and we choose theta to be 90 degrees because that's going to give us the maximum possible wavelength that will appear in the diffraction pattern because when you have, let's just consider two slits here for a second and you have a screen here and you have light going through these mini, mini slits in the diffraction grating and you're going to have a maximum appearing at the furthest possible angle being 90 degrees way up here. And this will maximize lambda because sine of 90 is the maximum sine you can possibly have which is one. This works out to 333 nanometers. So that's the maximum possible wavelength that'll show in the pattern and that is nevertheless shorter than the visible spectrum. So part c is asking us for the maximum number of lines on the diffraction grating. That would show a full second order maximum. So having a full second order maximum means that every wavelength in the visible spectrum will show up. And so we're going to choose lambda to be the longest wavelength of 700 nanometers. So the order is two. And so we have two times 700 nanometers written as times ten to the minus nine meters. And we divide this by sine 90 And we divide this by sine 90 because we're looking for just this threshold when it just barely shows up in the interference pattern. So that's 1.4 times ten to the minus six meters per line. And it asks us for the lines per centimeter. So we take the reciprocal that and say there's one line for every 1.4 times ten to the minus six meters and change the units into centimeters. And then that ends up being 7142 lines per centimeter.

