Question
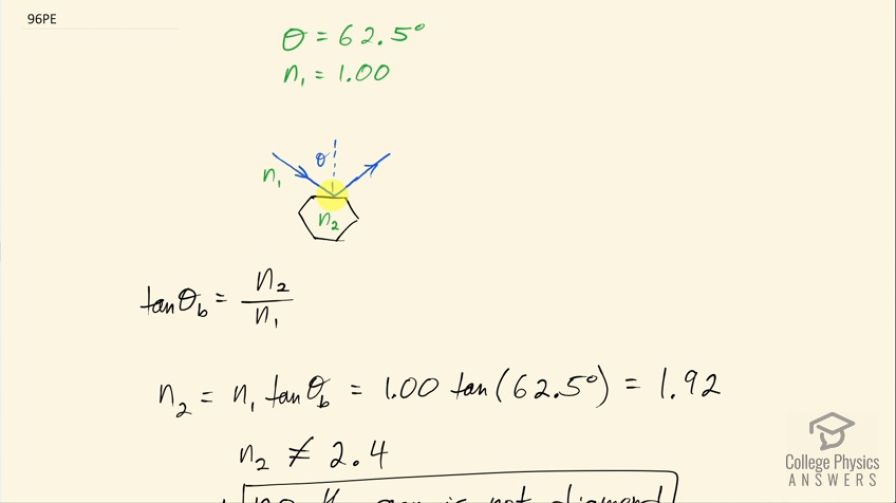
(a) Light reflected at from a gemstone in a ring is completely polarized. Can the gem be a diamond? (b) At what angle would the light be completely polarized if the gem was in water?
Final Answer
- No, the gem is not diamond.
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 96 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. When light is incident at an angle of 62.5 degrees and reflects off this gemstone, it is completely polarized and that means this angle is the Brewster's Angle and it's traveling in air initially so n 1 in this Brewster's Angle formula is the index of refraction of air and n 2 is the index of refraction of this type of gemstone. Now we are going to figure out what n 2 is and compare it with the index of refraction that we know that diamond has. So diamond has an index of refraction of 2.419 and so if our calculation gives us that number then we'll know it's diamond and otherwise, it is not diamond. So we will rearrange this to solve for n 2 by multiplying both sides by n 1 so the index of refraction of the gemstone is n 1 times tan of this Brewster's Angle so that's 1.00 times tangent of 62.5, which is 1.92 and that is not 2.4 so that means no, the gem is not diamond. Part (b) is asking suppose the light ray is traveling initially in water so this gemstone is submerged now and this n 1 is the index of refraction of water, what would the Brewster's Angle be in that case? So that means n 1 is 1.333 and we can look that up in table 25.1 for water here and we can take the inverse tan of both sides of this formula to solve for the Brewster's Angle: it's the inverse tan of n 2 over n 1. So that's the inverse tan of the index of refraction of the gemstone—1.921— divided by the index of refraction of the water—1.333— and that's 55.2 degrees.