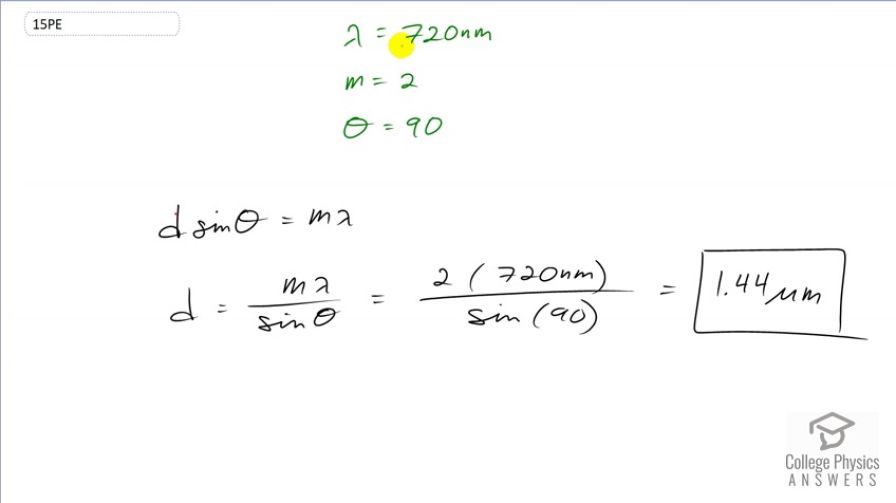
Question
What is the smallest separation between two slits that will produce a second-order maximum for 720-nm red light?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 27, Problem 15 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to find the smallest separation between slits that's possible giving a second order maximum for 720 nanometer light. So, the formula for maxima is separation between slits times sine of the angle equals the order times the wavelength. And, we'll divide both sides by sine Theta to solve for D. Now, we want the minimum here, and the only value we can really adjust on the righthand side of this equation is the angle because Lambda and M are both prescribed for us, 720 nanometers and two. And so, what value should Theta have in order to minimize D or in other words, maximize the denominator here? And, that will happen when Theta is 90. So, choosing 90 degrees for our angle will maximize sine and make it one and thereby minimize this quotient. So, we have two times 720 nanometers divided by sine of 90, which is 1.44 micrometers.
