OpenStax College Physics, Chapter 28, Problem 28 (Problems & Exercises)

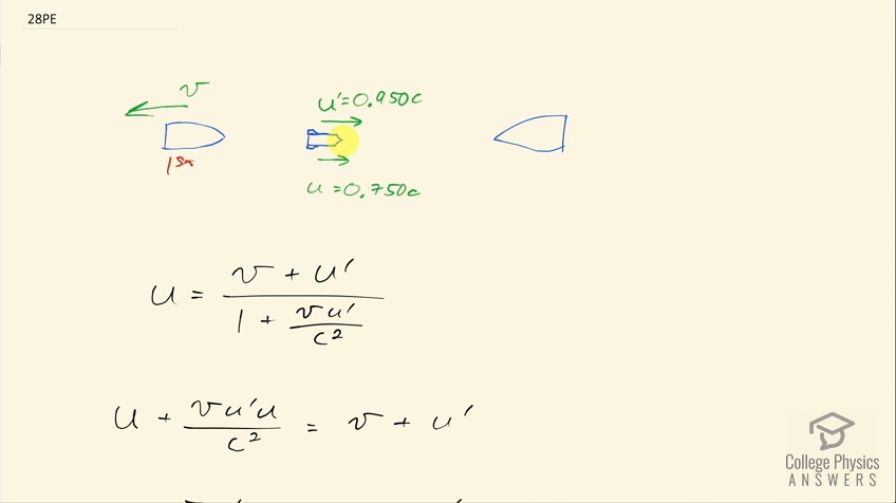
This is College Physics Answers with Shaun Dychko. A missile is shot from one spaceship towards another, the first spaceship reports that this missile is going away from it with a velocity of 0.950c; the second spaceship reports that the missile is approaching it with a velocity of 0.750c so in order to figure out whether these velocities should be labeled with u prime or u, it depends on which spaceship we have assigned the velocity v to. So I have decided to just put it on this first spaceship and it doesn't really matter which one you choose— the algebra's the same either way— so we'll take this to have velocity v in which case the first ships report for the velocity of the missile is going to be labeled u prime u prime always comes from the reference frame labeled with v. So u prime is 0.950c and that makes u 0.750c. So our formula is that u equals v plus u prime over 1 plus vu prime over c squared and we want to do some algebra to solve for v— the relative velocity of these two spaceships. We'll multiply both sides by 1 plus vu prime over c squared and then on the left side, after you multiply u into this binomial, we'll end up with u plus vu primeu over c squared and on the right hand side, we will have just v plus u prime. And then subtract v from both sides and also subtract u from both sides and we end up with vu primeu over c squared minus v equals u prime minus u. Then factor out the v from these two terms and then divide both sides by this binomial u primeu over c squared minus 1 and you have this formula here that v is u prime minus u over u primeu over c squared minus 1. So that's 0.950c is u prime minus 0.750c which was u divided by the product of those velocities over c squared minus 1 and that is negative 0.696c. So our answer is negative, which is to say that this first spaceship is moving to the left because we have to the right as positive since u prime and u are both positive and so our negative means that v is to the left and so these spaceships are moving apart and the ships are receding then away from each other with a relative velocity of 0.696c.