Question
What is the velocity of an electron that has a momentum of ? Note that you must calculate the velocity to at least four digits to see the difference from .
Final Answer
Solution video
OpenStax College Physics, Chapter 28, Problem 39 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
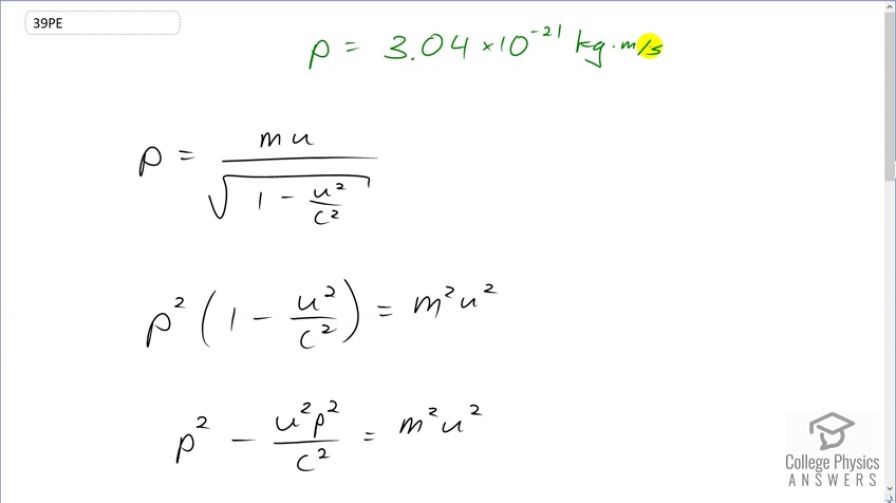
This is College Physics Answers with Shaun Dychko. The relativistic momentum of an electron is 3.04 times 10 to the minus 21 kilogram meters per second. and we are asked to figure out, what the velocity of the electron must be in order to have that momentum? So momentum is the mass of the electron times its velocity divided by square root of 1 minus its velocity squared divided by c squared and we have to do some algebra here to solve for u. So we'll square both sides which makes p squared and we have m squared u squared and then this square root becomes just a bracket, 1 minus u squared over c squared which we then multiply both sides by to end up in this next line, which is p squared times 1 minus u squared over c squared equals m squared u squared. And then distribute the p squared into the bracket and we have p squared minus u squared p squared over c squared equals m squared u squared. And then we want to collect the u terms together so we move this term to the right by adding it to both sides and then switch the sides around and we get m squared u squared plus u squared p squared over c squared equals p squared. Then factor out the u squared—common factor from these two terms. So u squared times m squared plus p squared over c squared equals p squared. And then divide both sides by this m squared plus p squared over c squared term and we end up with u after taking the square root of both sides equals p divided by square root of m squared plus p squared over c squared. And now we can plug in numbers. So the velocity of the electron is 3.04 times 10 to the minus 21 kilogram meters per second, which is the momentum and divide that by the square root of the mass of the electron, which is 9.11 times 10 to the minus 31 kilograms squared plus the momentum squared divided by speed of light squared, which gives a velocity of 2.9860 times 10 to the 8 meters per second.
