Question
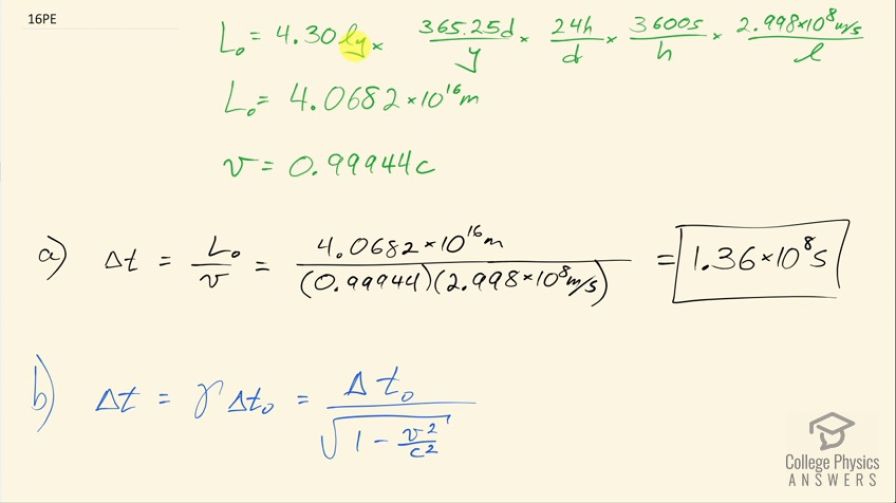
(a) How long does it take the astronaut in Example 28.2
to travel 4.30 ly at 0.99944c (as measured by the Earth-
bound observer)? (b) How long does it take according to the astronaut? (c) Verify that these two times are related through time dilation with as given.
Final Answer
- Yes, the two times are related by the Lorentz Factor.
Solution video
OpenStax College Physics, Chapter 28, Problem 16 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An observer on Earth measures the distance that an astronaut travels to be 4.30 light years in their trip to Alpha Centauri. We are going to convert light years into meters in order to use this distance in our calculation for time so I'll multiply by 365.25 days per year then multiply by 24 hours per day times 3600 seconds per hour and then multiply by 2.998 times 10 to the 8 meters per second for every, you know, speed of light I guess the l represents speed of light and a year is the time y and we are left with seconds times meters per second and this works out to meters. So the astronaut's traveling 4.0682 times 10 to the 16 meters according to an Earth-bound observer and this is going to be considered the proper length because the person on Earth is at rest with respect to the two end points that are being measured between the person on Earth is at rest with respect to Earth and also at rest with respect to Alpha Centauri— the destination— and so the distance they measure is proper length. The speed of the astronaut is 0.99944c, part (a) asks how long does it take for the astronaut to make this trip according to the Earth-bound observer? And that's going to be the distance that the Earth-bound observer measures divided by the speed and this is the time and it's not proper time because the person on Earth is not at rest with respect to the arrival and departure of the spaceship, it's the person that's on the ship that measures proper time. So this is Δt and that equals 4.0682 times 10 to the 16 meters divided by the speed, which is 0.99944 times the speed of light and this is 1.36 times 10 to the 8 seconds. Part (b) asks how long does it take according to the astronaut? Well, we know that the dilated time equals the Lorentz factor γ multiplied by proper time and the astronaut is going to be measuring proper time but first let's replace γ with 1 over square root 1 minus v squared over c squared and then we are going to solve for Δt naught so we multiply both sides by square root 1 minus v squared over c squared and then we end up with this line here because it cancels on the right and ends up being multiplied on the left and then switch the sides around and we get Δt naught is square root 1 minus v squared over c squared times Δt. So that's square root 1 minus 0.99944 squared times the time calculated in part (a) and this is 4.54 times 10 to the 6 seconds. And in part (c), we are asked to verify that the time measured by an Earth-bound observer is related to the time measured by the astronaut by this Lorentz factor and so Δt equals γ times Δt naught and so that's 30.00 times the answer to part (b) and this is 1.36 times 10 to the 8 seconds and sure enough that is the time we calculated in part (a) and so we have verified that yes, Δt and Δt naught are related by γ.