OpenStax College Physics, Chapter 28, Problem 19 (Problems & Exercises)

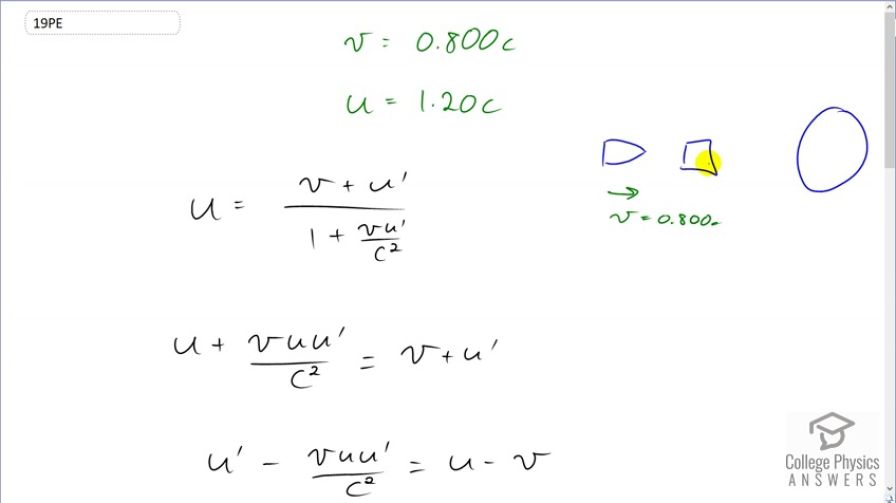
This is College Physics Answers with Shaun Dychko. A spaceship is moving towards the Earth with a speed of 0.800c. And the astronaut on the ship claims that they can fire a canister at some speed that would be measured at 1.20c by somebody on the Earth. So let's suppose that we are in the reference frame of the Earth and we see this canister coming towards us with a speed of 1.20c and the ship is moving towards us with this speed and so our question is, what speed will the astronaut on the ship measure for this canister? And they are going to be measuring u prime and so in this relativistic addition of velocities, we need to solve for u prime because u prime is measured by the observer that is moving with speed v, with respect to some other reference frame. OK. So, first, we'll multiply both sides by this denominator— 1 plus v u prime over c. And then we end up with u plus vu u prime over c squared; there's a squared on the bottom there and that equals v plus u prime, on the right hand side because these two cancel. And then we want to collect the u prime terms together so we'll subtract this term from both sides so we are moving it to the right hand side, in other words. And we'll subtract the v from both sides and then we'll switch the sides around. And we have u prime minus v u u prime over c squared equals u minus v and this is useful because we have the u prime factor in both of these terms and then we can factor it out. So you have u prime times 1 minus vu over c squared equals u minus v. And then we'll divide both sides by this bracket is 1 minus vu over c squared and then we solve for u prime. So u prime then is u minus v over 1 minus vu over c squared. So, u is the velocity measured by the Earth based observer; v is the velocity with which the spacecraft is approaching the Earth, and u prime is the velocity of the canister measured by the astronaut which is in this moving reference frame. So we have 1.20c is the alleged speed of the canister, measured by somebody on Earth minus 0.80c, speed that the ship is approaching the Earth divided by 1 minus 0.80c times 1.20c over c squared and this gives 10 times c. Now, that is very impossible because nothing can go faster than the speed of c and it's unreasonable to presume that this canister will approach the Earth with a velocity of 1.20c. It's unreasonable to think that it will approach the Earth at any speed greater than c.