Question
(a) Calculate the speed of a particle of dust that has the same momentum as a proton moving at . (b) What does the small speed tell us about the mass of a proton compared to even a tiny amount of macroscopic matter?
Final Answer
- This small velocity of the dust particle says the proton has a very significantly smaller mass than the dust.
Solution video
OpenStax College Physics, Chapter 28, Problem 41 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
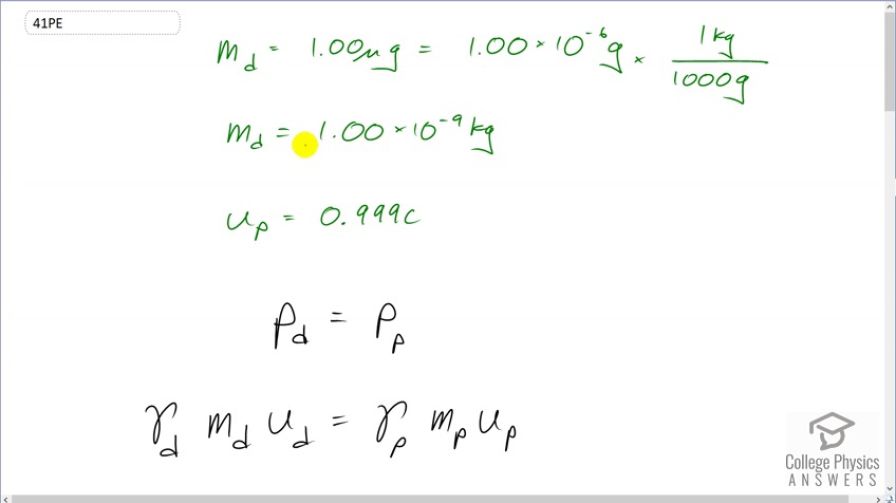
This is College Physics Answers with Shaun Dychko. We are given the velocity of a proton which is 0.999c and we are given the mass of a speck of dust which is 1 microgram which we convert into kilograms by multiplying 1 times 10 to the minus 6 grams by 1 kilogram for every 1000 grams giving us 1 nanokilogram, well which is kind of a funny way of saying things, two prefixes together but anyway, it's 1 times 10 to the minus 9 kilograms. And we write it this way because we always want meters, kilograms and seconds in our units in our formulas, mks units. OK. So we are told that the momentum of the dust particle is the same as the momentum of the proton and with that being the case, what must the velocity of the dust particle be? So we have the relativistic momentum of the dust particle is the Lorentz factor times the mass of the dust particle times its velocity and that equals the Lorentz factor of the proton times the proton's mass times the velocity of the proton. And then we substitute for the Lorentz factor, in both cases here and then I'm gonna plug in numbers to figure out what is the momentum of the proton. So we have the mass of the proton— 1.673 times 10 to the minus 27 kilograms— times its velocity which is 0.999 times the speed of light and divide that by square root of 1 minus 0.999 squared because this 0.999 is the factor times c which then gets squared and then divided by c squared and the c squared's canceled. And this works out to 1.12069 times 10 to the minus 17 kilograms meters per second; that's the momentum of a proton. And that is going to be equal to the momentum of the dust particle. And now we have to solve this for the velocity of the dust particle. We'll begin by squaring both sides and that gives us m d squared u d squared over 1 minus u d squared over c squared equals the momentum of the proton squared. And then we'll multiply both sides by this denominator here so both sides get multiplied by 1 minus velocity of the dust particle squared over c squared. And on the left side that just cancels away and we are left with the numerator here. And on the right side, we'll distribute the momentum of the proton into this bracket and so we have momentum of the proton squared minus velocity of the dust particle squared times the momentum of the proton squared over c squared. Then we'll collect the terms containing the factor u d on the same side and so this term gets moved to the left and then factor out the u d squared from both terms and we are left with u d squared times mass of the dust particle squared plus momentum of the proton squared over c squared equals this momentum of the proton squared which is left over on the right hand side. Then divide both sides by this bracket here and then take the square root of both sides and we are left with the velocity of the dust particle equals the momentum of the proton divided by the square root of the mass of the dust particle squared plus momentum of the proton squared over c squared and now we can plug in numbers. So this is our momentum of the proton that we calculated before divided by the square root of the mass of the dust particle which is 1 times 10 to the minus 9 kilograms squared plus momentum of the proton squared divided by speed of light squared and that gives 1.12 times 10 to the minus 8 meters per second. That is a very very very small speed and so that shows that despite the proton's very high speed, the proton's small mass compensates for that high speed such that the momentum of the proton is really small. And so the dust particle needs a mere 1.12 times 10 to the minus 8 meters per second of velocity in order to have the same momentum as that very fast proton which says that the mass of the proton is very significantly smaller than the mass of the dust.
