Question
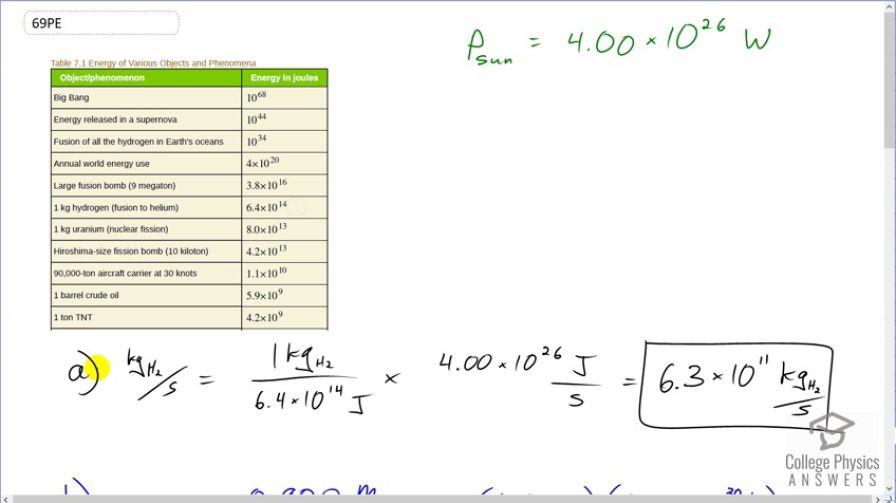
The Sun produces energy at a rate of by the fusion of hydrogen. (a) How many kilograms of hydrogen undergo fusion each second? (b) If the Sun is 90.0% hydrogen and half of this can undergo fusion before the Sun changes character, how long could it produce energy at its current rate? (c) How many kilograms of mass is the Sun losing per second? (d) What fraction of its mass will it have lost in the time found in part (b)?
Final Answer
Solution video
OpenStax College Physics, Chapter 28, Problem 69 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
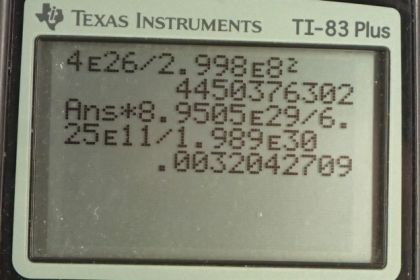
This is College Physics Answers with Shaun Dychko. The Sun is producing energy at a rate of 4.00 times 10 to the 26 watts and part (a) asks us, how many kilograms of hydrogen undergo fusion every second? So from this data table from chapter 7, table 7.1, we know that when 1 kilogram of hydrogen undergoes fusion, it produces 6.4 times 10 to the 14 joules of energy. So we want to know how many kilograms of hydrogen undergo fusion per second in the Sun. So we are going to multiply 1 kilogram for every 6.4 times 10 to the 14 joules; that's expressing this piece of data as a fraction and I have written it this way so that we have kilograms on top because we want to know kilograms per second and are the units of our answer. So we have a kilogram for this many joules times 4.00 times 10 to the 26 joules every second— this is the energy output of the Sun— and the joules cancel leaving us with with kilograms per second. So 6.3 times 10 to the 11 kilograms of hydrogen undergo fusion every second. Then in part (b), we are asked for how long will this fusion carry on before the Sun changes its character? Now, we are told that 90 percent of the Sun is hydrogen and so we can figure out, how much hydrogen there is by going 0.900 times mass of the Sun. So that's 0.900 times 1.989 times 10 to the 30 kilograms and divide that by 2 to get this much mass of hydrogen that's available for fusion before the Sun changes its character. And we know that in every second, there are 6.25 times 10 to the 11 kilograms of hydrogen undergoing fusion; this is our answer for part (a) which I have you know, expressed with three significant figures instead of only two since this is an intermediate calculation. And so for every second, we have this many kilograms fusing multiplied by this many kilograms of hydrogen that are available and then this would give us the number of seconds that the Sun can do fusion for but let's express this in years because we can understand years a bit better although this number is so big, it's hard to understand anyway. But we'll multiply this by 1 hour for every 3600 seconds times 1 day for every 24 hours times 1 year for every 365.25 days giving us years. So this is 4.5 times 10 to the 10 years which is 45 billion years which is much longer than the current age of the Earth and so on. So that's a very long period of time. Part (c) is how much mass is being lost by the Sun since it's being converted into energy? Now, the answer for part (a), by the way, this is the mass of hydrogen that undergoes fusion but most of this mass is not lost, most of it is turned into mass of the helium that remains, after the fusion. But there is some mass that is turned into energy and that's the answer for part (c). So the amount of energy produced is gonna be the amount of mass lost, or converted, times c squared and we'll solve for Δm by dividing both sides by c squared and then switching the sides around. So the amount of mass lost is the amount of energy divided by c squared and the energy produced is the power output multiplied by time. So we can substitute pt in place of E in this formula for Δm. So we have the power output of the Sun is 4.00 times 10 to the 26 joules per second and we are told to say how much mass is lost every second and so that means our time is 1 second because the question told us in 1 second, how much mass is turned into energy? And we divide by the speed of light squared and we get 4.5 times 10 to the 9 kilograms. So four and a half billion kilograms of hydrogen are turned into energy every second. And then in part (d) says after this total time period of 4.5 times 10 to the 10 years, what fraction of the Sun's mass will have been turned into energy? And so the amount of mass that's turned into energy is Δm and this denominator m is the initial total mass of the Sun. So this denominator, m, we look up in the data table—mass of the Sun. And the numerator is going to 4.45 times 10 to the 9 kilograms that are turned into energy every second, which is our answer for part (c), multiplied by the number of seconds that the Sun will be doing this fusion for before it changes character. Now I could have taken 4.5 times 10 to the 10 years and converted it into seconds and written that here, that would be fine, but what I have done instead just to have less writing is to take the amount of hydrogen that is available for fusion and then divide by the rate at which it is fusing and that's the answer for part (a); divide by 6.25 times 10 to the 11 kilograms for every second. So this gives the number of seconds during which the Sun will be doing fusion and then times by this rate of energy conversion in or mass conversion into energy. Then divide by the original mass of the Sun and we get 0.32 percent which is surprisingly small actually because, you know, this is a huge amount of mass that's being lost and turned into energy every second but in comparison to the absolutely astronomically huge mass of the Sun, this represents a small percentage.