Question
Two planets are on a collision course, heading directly towards each other at . A spaceship sent from one planet approaches the second at as seen by the second planet. What is the velocity of the ship relative to the first planet?
Final Answer
Solution video
OpenStax College Physics, Chapter 28, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
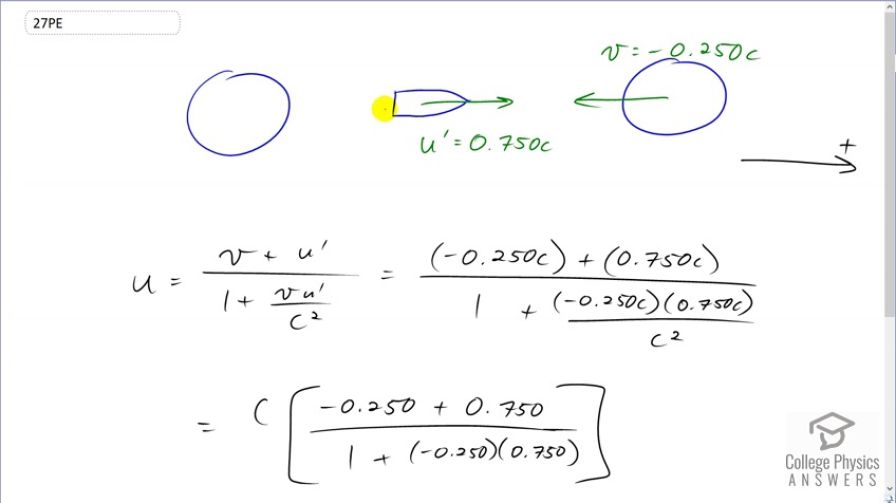
This is College Physics Answers with Shaun Dychko. We know that two planets are approaching each other with a speed of 0.250c and that the velocity of this spaceship is being measured as 0.750c towards one of them. And so the question is, which planet should get labeled with this velocity v? Well, I have chosen it to be this one because I want to use this relativistic addition of velocities formula in a way that I don't have to rearrange it, I don't want to do any algebra to solve for u prime; I'd rather just plug in numbers and get u. So I can do that if I choose to have this planet be the one that's moving to the left and which makes it have a velocity of negative 0.250c since I have chosen a coordinate system with positive to the right. And by choosing this planet to be the one that is the moving reference frame that makes this velocity, which this planet measures, 0.750c; it makes it u prime because u prime is measured by the observer in the reference frame that we chose and to consider moving. And in that case, the reference frame of this observer is at rest and we'll say that this one is the one that's moving—0.250c— negative because it's to the left and that this velocity of the spaceship towards this moving planet is gonna be u prime. OK. So now we plug in numbers into this formula to get our answer for u, the velocity of this spacecraft according to somebody on this other planet. So we have negative 0.250c, velocity of this moving reference frame. plus 0.750c positive because this spacecraft is moving to the right and we divide that by 1 plus negative 0.250c times 0.750c over c squared and this works out to c times negative 0.250 plus 0.750 divided by 1 plus negative 0.250 times 0.750 because these c's got factored out there. And then this works out to 0.615c and we expected this answer to be less than 0.750 since this is a number that's being reported by an observer on this planet and since this planet is approaching this spacecraft, they are going to be reporting a velocity that is combining this approach velocity with the observer's own velocity to the left. And so we expected the observer here to be measuring only the approach velocity, so to speak; I mean it's not quite that simple but it's a good way to think of it. So anyway, in any case, we expected this to be less than 0.750c, which it is, and it is to the right because it's positive.
