Question
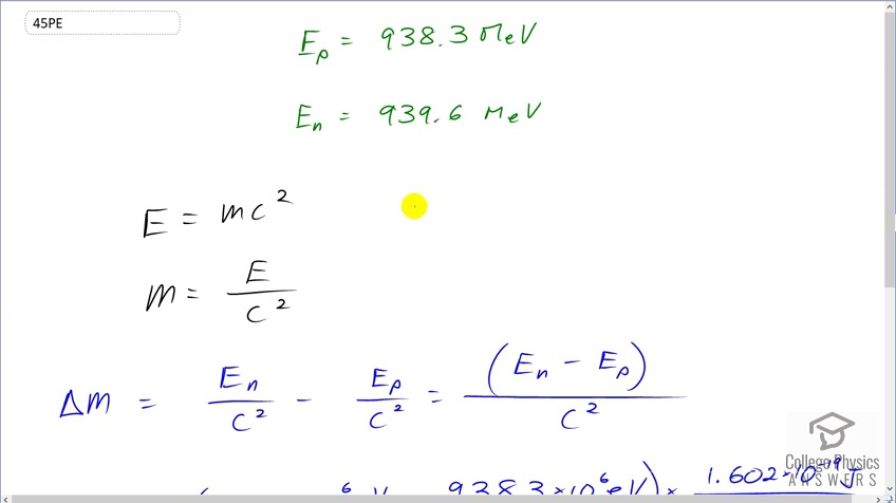
If the rest energies of a proton and a neutron (the two constituents of nuclei) are 938.3 and 939.6 MeV respectively, what is the difference in their masses in kilograms?
Final Answer
Solution video
OpenStax College Physics, Chapter 28, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The rest energy of a proton is 938.3 megaelectron volts and the rest energy of a neutron 939.6 megaelectron volts and this question asks us to find the difference in mass of these two sub-atomic particles, in kilograms. So rest energy is mass times speed of light squared and we can solve this for m by dividing both sides by c squared then switching the sides around. We have m equals the energy divided by c squared. So that means the difference in mass between these two particles is gonna be the difference in, well, using this formula; energy of the neutron over c squared minus rest energy of the proton over c squared. And then we can at least have a common factor, or a common denominator, I should say. And so we can write it as E n minus E p over c squared. So the difference in rest energies of the neutron and proton can be multiplied by 1.602 times 10 to the minus 19 joules for every electron volt and this megaelectron volts, I wrote as times 10 to the 6 electron volts. And then this difference gets multiplied by this conversion factor to turn it into joules and that means when we divide by the speed of light squared, our answer will be in kilograms. And so we have 2.32 times 10 to the minus 30 kilograms is the mass difference between a neutron and a proton.
