Question
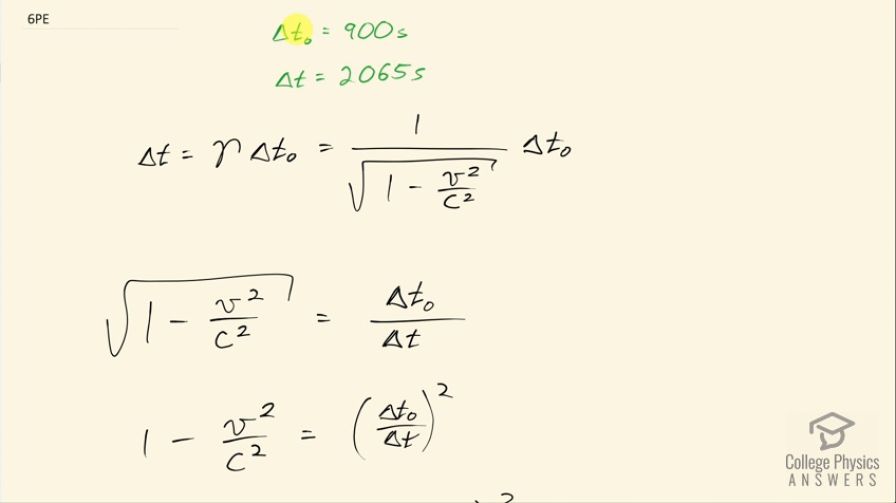
A neutron lives 900 s when at rest relative to an observer. How fast is the neutron moving relative to an observer who measures its life span to be 2065 s?
Final Answer
Solution video
OpenStax College Physics, Chapter 28, Problem 6 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A neutron at rest with respect to an observer has a lifetime of 900 seconds so this time is called t naught— the proper time— since it's measured at rest with respect to the neutron; in a different experiment when the neutron is zipping past the observer, its lifetime is measured to be 2065 seconds and this Δt—the time measured by someone watching it zip past— equals the Lorentz factor γ times the proper time and we will use this relation to figure out the speed with which the particle is moving in this scenario here. So γ is 1 over the square root of 1 minus v squared over c squared times Δt naught and we have to do some algebra to solve for v. So first we'll multiply both sides by this Lorentz factor 1 minus v squared over c squared and we'll also divide both sides by Δt. So we have square root 1 minus v squared over c squared equals Δt naught over Δt then we square both sides and I have 1 minus v squared over c squared equals Δt naught over Δt squared and then add v squared over c squared to both sides and then subtract Δt naught over Δt squared from both sides and then switch the sides around and we have v squared over c squared equals 1 minus Δt naught over Δt squared then multiply both sides by c squared and then you get this line here and take the square root of both sides to finally solve for v. So v then is c multiplied by the square root of 1 minus Δt naught over Δt squared. So that's 2.998 times 10 to the 8 meters per second times the square root of 1 minus 900 seconds divided by 2065 seconds squared and this is 2.70 times 10 to the 8 meters per second.

