Question
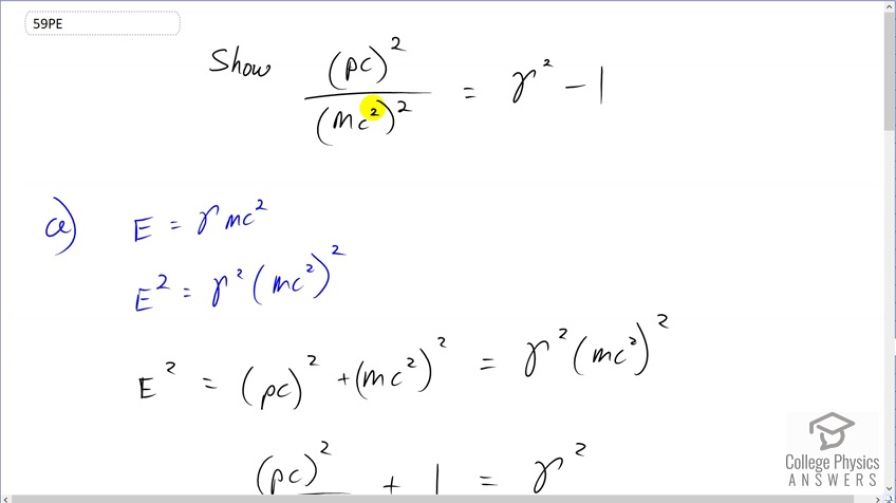
(a) Show that . This means that at large velocities . (b) Is when , as for the astronaut discussed in the twin paradox?
Final Answer
Please see the solution video.
Solution video
OpenStax College Physics, Chapter 28, Problem 59 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to show that momentum times speed of light squared divided by mass times speed of light squared, all squared is going to equal the Lorentz factor, gamma, squared minus 1. So we have an expression here for the total energy of a particle squared equals pc squared plus mc squared squared and this looks like a good thing to use since it has a lot of terms in it that are look the same as what we are working with here; this pc squared term shows up and this mc squared squared term shows up here. So we just start with that and then we'll find another expression for it, in terms of gamma, by looking at this which is to say that total energy is Lorentz factor times mc squared. And then the reason I squared it, on both sides, is so that we create factors that resemble what we are working with here. So by squaring both sides of this, we end up with energy squared equals gamma squared times mc squared squared. So we set this equal to E squared here. And now we can divide both sides by mc squared squared and then that turns this into a 1 and we have this fraction plus 1 equals gamma squared and then subtract 1 from both sides and we are done. We have shown that pc squared over mc squared squared equals gamma squared minus 1. Then in part (b), we are asked is energy approximately equal to momentum times speed of light, when gamma is 30? And so, in other words, is the total energy just a function of momentum and the rest energy can be ignored? This is rest energy in here and it's squared and when it reaches some high speed, this rest energy will be small in comparison to the momentum momentum times speed of light squared. OK. So we know this formula for total energy squared and that's given to us in the textbook and then we can substitute for mc squared squared with this expression which contains pc in it and it's useful to do this substitution because we want to equate energy approximately to pc so on the right hand side, we wanna have just those variables, momentum and speed of light, if we can. And gamma is fine because we are told gamma is 30 and this expression came from a rearrangement of this here because if you multiply both sides by this denominator and then divide both sides by this bracket, you will end up with this expression. So mc squared squared equals pc all squared over gamma squared minus 1. So we substitute this in place of mc squared squared here and that's why I have written this in red. So then we factor out the pc squared from both terms here and we have energy squared then is pc squared times bracket 1 plus 1 over gamma squared minus 1. And then we can take the square root of both sides and then we have energy equals pc times this square root here—1 plus 1 over gamma squared minus 1. Now, if we can show that the square root is approximately 1 then we will have shown that E bascially equals pc. So let's substitute in the number 30 for gamma and we have E equals pc times square root 1 plus 1 over 30 squared minus 1 and we end up with square root of 1 plus 0.00111 and the square root of 1.00111 is 1.0006. And so this is approximately 1 to 4 significant figures and so we can say, yes, when gamma is 30 the energy approximately is the same as the product of momentum and the speed of light.