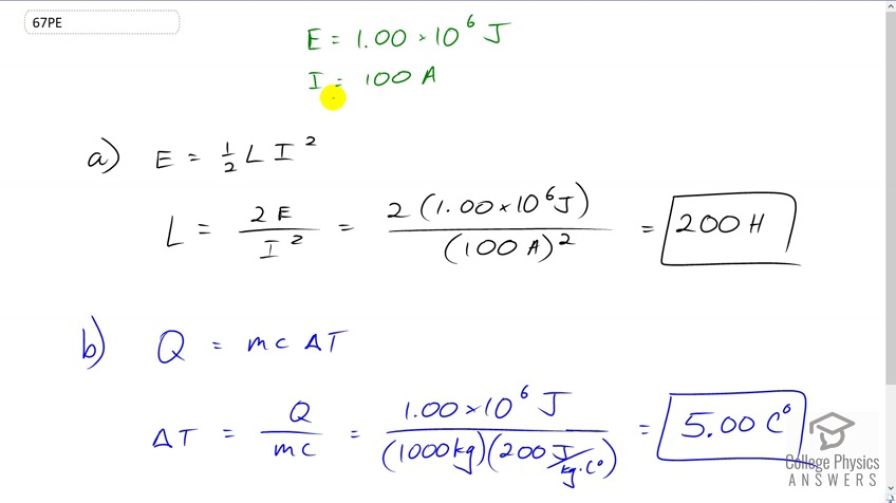
A very large, superconducting solenoid such as one used in MRI scans, stores 1.00 MJ of energy in its magnetic field when 100 A flows. (a) Find its self-inductance. (b) If the coils “go normal,” they gain resistance and start to dissipate thermal energy. What temperature increase is produced if all the stored energy goes into heating the 1000 kg magnet, given its average specific heat is $200\text{ J/kg}\cdot {\text{C}}^{\circ }$?

Video Transcript

This is College Physics Answers with Shaun Dychko. A super-conducting MRI magnet stores one megajoule of energy which is 1 times 10 to the 6 joules and has a current of 100 amps and the question is, what is the inductance in this magnet? So, we will solve this formula for L. So, energy is one-half times inductance times current squared and we will solve for L by multiplying by 2 over current squared on both sides and this gives us L is 2 times energy divided by current squared. So, that’s 2 times 1 times 10 to the 6 joules divided by 100 amps squared giving us an inductance of 200 henrys. Then part B says suppose the super-conducting magnet goes normal in which case it's not super-conducting anymore; what will its change in temperature be giving that all its energy stored in it turns into thermal energy? So, we have the amount of heat energy added to something is gonna equal its mass times its specific heat times its change in temperature and we will solve for delta T by dividing both sides by m c and then switch the sides around. So, the change in temperature is the heat energy divided by mass times specific heat and we are given the mass is 1000 kilograms and specific heat of this magnet material is 200 joules per kilogram per Celsius degree and we divide that into 1 megajoule and that makes a temperature change of 5 Celsius degrees.