Question
A 75-turn, 10.0 cm diameter coil rotates at an angular velocity of 8.00 rad/s in a 1.25 T field, starting with the plane of the coil parallel to the field. (a) What is the peak emf? (b) At what time is the peak emf first reached? (c) At what time is the emf first at its most negative? (d) What is the period of the AC voltage output?
Final Answer
- Peak EMF first occurs at time , when the generator is first turned on.
- The next peak EMF occurs at
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 36 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
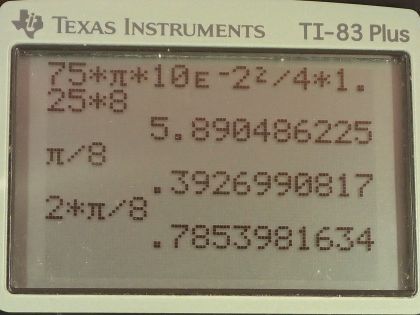
This is College Physics Answers with Shaun Dychko. A circular coil of diameter 10.0 centimeters and 75 turns of wire rotates at a speed of 8.00 radians per second in a 1.25 tesla magnetic field and the question in part (a) is what is the peak emf that is going to be generated? So peak emf is the number of turns multiplied by the area of the coil times the magnetic field strength times the angular speed. So the area is π times radius squared but we are given the diameter so we divide that by 2 to substitute for radius and then this works out to π times diameter squared over 4. So we substitute that in place of A and then the peak voltage then is 75 turns times π times 10.0 times 10 to the minus 2 meters squared over 4 times 1.25 tesla times 8.00 radians per second which is 5.89 volts. Part (b) is asking at what time is the first peak emf reached? So initially we are told that the loop has its plane parallel to the magnetic field lines or the angle Θ in this formula for flux, which is magnetic field times area times cos Θ; Θ is typically measured as the angle between the perpendicular to the loop plane and the magnetic field. So while the angle between the plane and the magnetic field is zero here, the angle between the perpendicular to the plane and the magnetic field is 90 degrees, or in radians, it's π over 2 radians. A quarter of a turn later—supposing it's rotating this direction— the plane will be perpendicular to the magnetic field or the normal to the plane is parallel to the magnetic field in which case, the angle is 0 or you might call it, π radians I am gonna be talking about a graph down here so I think in terms of... let's just call it zero... that makes more sense we are just measuring the acute angle that's between this normal to the plane the word normal here is the word meaning perpendicular this perpendicular line has a zero degree or zero radian angle between it and the magnetic field... okay... all this to say that the flux is maximum after a quarter of a turn. So the emf that's generated in the loop is the rate of change of this flux and so this is why we are interested in the flux because the rate of change of it is going to help us figure out when the initial peak emf occurs. I have a data table here with some sort of... not really like I didn't put numbers here, I am not doing calculations of what the flux is— that's not important— the important thing is when does it occur at a maximum or minimum? So I am gonna make a graph of flux versus time and then if you think back to your kinematics work, if you had a graph of position versus time and if you were to take the slope of this graph the slope of it would be the speed and so in a same way, we are going to analyze this graph— this is flux versus time— and if we find the slope of the flux versus time graph that slope is the rate of change of flux, in other words, the emf so this is one way to look at it. So let's see how we built this graph: we have at time 0, the angle is π over 2 or 90 degrees and the flux is zero because there are no field lines going through the loop and after a quarter of a turn, the flux is well... minimum or maximum depending on how you look at it negative or positive this is the moment when the flux is of greatest magnitude but negatively speaking, we will call it a minimum and that's what happens here— that's this point here on the graph— and then after a half turn, the loop will then be parallel again to the magnetic field lines so the loop would be like this and the perpendicular would be like that and the field lines would be here and this is a half turn later... I think you get the idea and there will be no flux in that case. And then at three quarter of a turn, this normal would be facing down— maximum flux— and then after a full time period has passed, the period being the time it takes to do one complete rotation that after one full period, it's back to this picture and there's π over 2 angle between the normal and the magnetic field lines in which case the flux is 0. Okay! So we can make a flux versus position graph but I wanted to make a flux versus time graph because this connects more with this equation since we want to know the rate of change of the flux with respect to time. So the rate of change of a graph at a single moment in time is the slope of a tangent line there and so we can see here the slope is of greatest magnitude; here it's a very large magnitude negative slope and here it's a very large magnitude positive slope (oops) if you don't like looking at it that way, you could instead say consider a given interval of time like from here to here however much time that is it doesn't matter what the number is in seconds but it's some some sort of distance horizontally on our graph compare how much the flux changes in this given time and so we can see that the flux will change from zero to whatever this number is here— this big change— and take that same interval of time and begin at this negative peak here after one quarter period and then moving from here to here horizontally the exact same amount and we are gonna compare how much the flux changes on the vertical axis and it goes from here to here— it barely changes at all— so this is a small change. This is a different way of saying the same thing as I was trying to say with the slope of the tangent line in that the flux will change a lot for a given amount of time at this moment—time zero— and also at time one-half period but the flux will change only a small amount indicated by this small vertical change on the flux axis in the same amount of time when you begin at this time of one quarter period or three quarters period. So the very first time at which the rate of change of flux is greatest is at the beginning at time zero when the generator is first turned on so that's the answer for part (b). Part (c) asks for the next peak voltage and that occurs at one-half period and so we had better figure out what the period is because it's gonna ask for an answer in seconds so we have one complete rotation, which is 2π radians divided by 8.00 radians per second— this is the angular speed— and that is 0.785398 seconds and half of that is 0.393 seconds so this will be the time of the next peak voltage. And of course the answer for (d) based on the work we did for (c) is 0.785 seconds is the period or the time to do one complete rotation.