Question
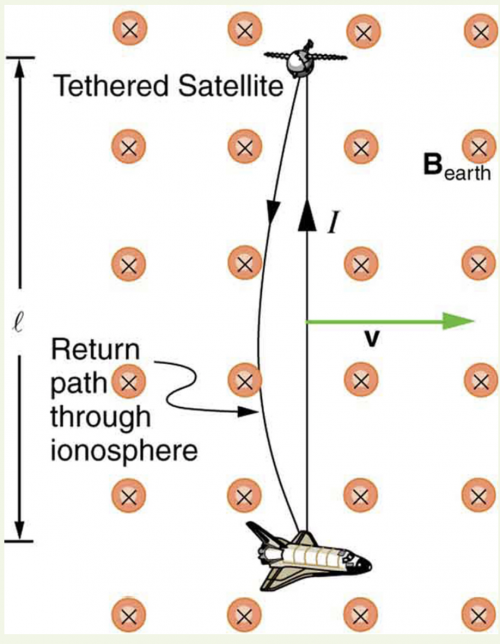
The Tethered Satellite in Figure 23.12 has a mass of 525 kg and is at the end of a 20.0 km long, 2.50 mm diameter cable with the tensile strength of steel. (a) How much does the cable stretch if a 100 N force is exerted to pull the satellite in? (Assume the satellite and shuttle are at the same altitude above the Earth.) (b) What is the effective force constant of the cable? (c) How much energy is stored in it when stretched by the 100 N force?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 24 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In this tethered satellite experiment, we have the space shuttle of mass 525 kilograms with a tether that is 20.0 kilometers long attached to this satellite; the diameter of this cable is 2.50 millimeters and we are told that the cable has the tensile strength of steel and so we need to look up Young's modulus from chapter 5 and for steel, it's 210 times 10 to the 9 newtons per square meter. The force exerted on this cable is 100 newtons and the question we are asked is how much will the cable stretch? So the change in length of this wire is gonna be 1 divided by the Young's Modulus multiplied by the force on it divided by its cross-sectional area multiplied by its original length— this is from chapter 5 this formula. So we can get the cross-sectional area because it's a circular cross-section... it's a cylindrical wire in other words so the area is going to be π times radius squared but we are not given the radius, we are given the diameter so we have to divide that diameter by 2 to substitute for radius and we end up with π times diameter squared over 4 is our area and we can substitute that in here and when dividing by this fraction, we can instead multiply by its reciprocal so I am multiplying by 4 over πd squared. And so we have 1 over 210 times 10 to the 9 newtons per square meter times 4 times 100 newtons divided by π times the diameter squared times the original length written as 20.0 times 10 to the 3 meters and we have 1.94 meters of stretching. In part (b) we are asked what is the effective force constant and this is also called the Hooke's constant in Hooke's Law so this is the elastic force that the thing being stretched exerts and it's in the opposite direction to the change in length and we can solve this for k. So we divide both sides by Δx— the amount of stretching— and we get k then is the force divided by Δx. Now this negative sign is not important, it's just to remind us that the force direction is opposite to the stretching direction; we are just gonna take the absolute value of all this and so we have absolute value of negative hundred divided by 1.94 meters of stretching and that's 51.5 newtons per meter is the force constant. The potential energy stored in this stretched cable is one-half times this force constant times the amount of stretching squared so that's one-half times 51.5464 newtons per meter times 1.9402 meters squared and that's 97.0 joules.
