Question
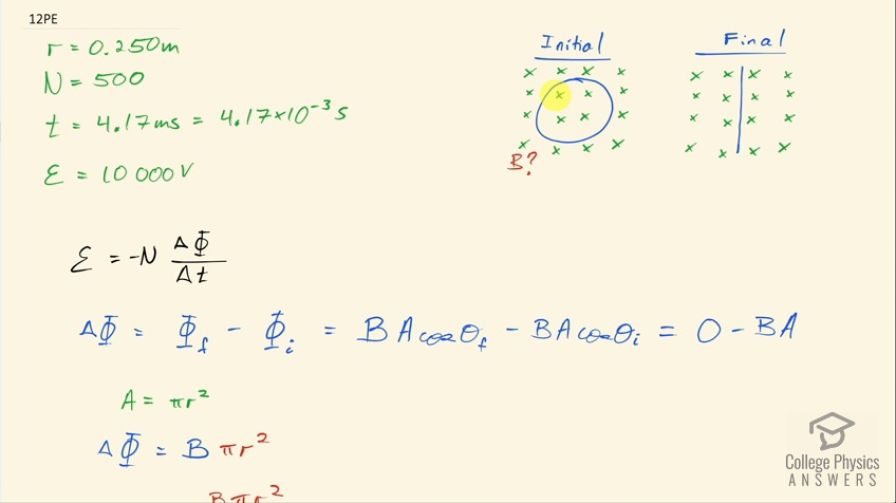
A 0.250 m radius, 500-turn coil is rotated one-fourth of a revolution in 4.17 ms, originally having its plane perpendicular to a uniform magnetic field. (This is 60 rev/s.) Find the magnetic field strength needed to induce an average emf of 10,000 V.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 12 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A loop with 500 turns of wire initially has a plane perpendicular to the magnetic field and then this loop will be rotated a quarter turn in 4.17 milliseconds and then the induced emf we are told is meant to be 10000 volts so what should the magnetic field strength be to make that happen and we are given that the loop's radius is 0.250 meters. So the induced emf is the number of turns of wire multiplied by the rate of change in flux so this is the change in flux divided by the amount of time it takes to make that change. The change in flux then is the final flux minus the initial flux; the final flux is the magnetic field strength multiplied by the area of the loop times cosine of the final angle minus the magnetic field strength times the area times cos of the initial angle. So the initial angle is 0 degrees because the angle is the angle between the perpendicular to the plane of the loop and the magnetic field so the perpendicular to the plane of the loop is coming right out of the page or into the page— depending how you want to look at it— and it's parallel to the magnetic field and so the angle is zero initially. And then the final state has the plane perpendicular to the plane of the loop perpendicular to the magnetic field lines and so there's no flux in this final orientation of the loop. Another way to say it is that there are no magnetic field lines passing through the loop; that's another way of saying there's no flux. So we have 0 for our final flux minus BA for the initial flux. The area is π times radius squared so we plug that in for A and so our change in flux then is Bπr squared. And I guess I could put a negative sign there: the negative's here are not so important, they just remind us that... like this negative reminds us that the induced emf is in a direction such that the induced magnetic field opposes the change in flux but really, you should be getting that direction by looking at the situation and using the right hand rule. But nevertheless, you can plug a negative in here and a negative there and it ends up being positive so the induced emf then is number of turns times magnetic field strength times π times radius squared over change in time and we have to solve this for B by multiplying both sides by both sides by Δt over Nπr squared. So then the magnetic field strength is the induced emf times Δt over Nπr squared because these things cancel over here and we have 10000 volts times 4.17 times 10 to the minus 3 seconds divided by 500 turns times π times one quarter meter radius squared and that is 0.425 tesla is the required magnetic field strength.
