OpenStax College Physics for AP® Courses, Chapter 23, Problem 4 (Test Prep for AP® Courses)

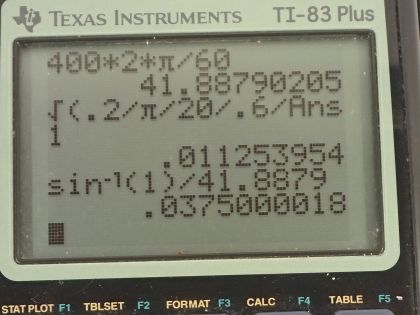
This is College Physics Answers with Shaun Dychko. A coil that has a circular cross-section has 20 turns and it's rotating at a rate of 400 rpm which I am writing as revolutions per minute because I want to convert this into radians per second since radians per second is an mks unit— meters, kilograms and seconds— and might as well take care of this unit conversion at this stage where we are just writing down the things that we know rather than being burdened by that conversion later when we are thinking about other stuff. So 400 revolutions per minute times 2π radians in every revolution then multiply by 1 minute for every 60 seconds gives us radians per second here and this works out to 41.8879 radians per second. The magnetic field strength in which this coil is rotating is 0.6 tesla and there's a peak induced emf of 0.2 volts. So the first question is what is the radius of the coil? The peak emf is the number of turns times the cross-sectional area times the magnetic field strength times the rotational speed— angular velocity in other words— so the area since it's a circular cross-section is π times r squared so we substitute that in place of A and then solve for r squared by dividing both sides by πNBω and we have r squared is peak voltage divided by π times number of turns times magnetic field strength times angular velocity and then take the square root of both sides. So r then is the square root of 0.2 volts divided by π times 20 turns times 0.6 tesla times 41.8879 radians per second and this works out to 11 millimeters. Part (b) says supposing that the emf in the coil is 0 at time equals 0 which means that we can use this formula, which is that the emf induced is the peak emf times sin ωt and there's no need for a term in this bracket to add some sort of phase difference, you know because it's 0 at time equals 0 and sin of 0 is 0 so that works nicely and we want to know at what time will the peak emf be induced? And so that means this side here is E naught as well so we have to solve this for t. So we'll divide both sides by the peak emf and we have sin ωt then is 1 because emf peak divided by emf peak is 1 and then take the inverse sign of both sides and we have ωt equals the inverse sin of the number 1 and divide both sides by the angular velocity and we have time is inverse sin of 1 divided by ω. So that's inverse sin of 1 divided by 41.8879 radians per second and if you don't get this answer, it's because it's very important to have your calculator in radian mode and different calculators will require different button pushes— who knows where—in order to make that change but it will either have a radian mode or a degree mode and for the purposes of this calculation, you need to have your answer in radian mode because our denominator is in units of radians per second; if we had degrees per second then I suppose you could have your calculator in degree mode but we have radians per second so the calculator needs to be in radian mode. That is 0.038 seconds is when the peak emf will first occur.