Question
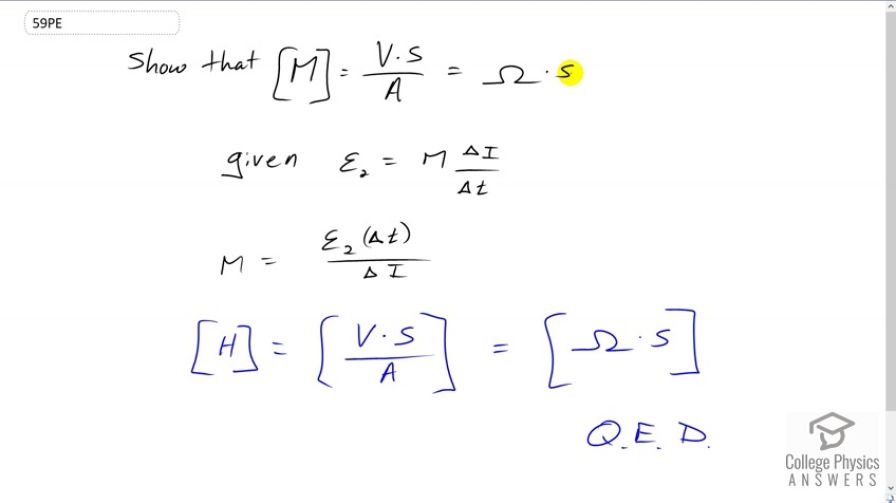
Starting with , show that the units of inductance are
Final Answer
Please see the dimensional analysis in the solution video.
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 59 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We are going to show that the unit of inductance are volt second per amp or ohm second. So, we have this formula that tells us the voltage is inductance times rate of change of current and then we can solve this for M and say that its emf induced times the amount of time divided by change in current and we do that by multiplying both sides by delta t over delta I and then we can look at the units we are left with here. So, this emf has unit of voltage, this time has unit of second and this current has unit of amp and this inductance here, mutual inductance has units of henry. That’s what we are going to show the unit of henry is volts second per amp and so we showed that already because that’s the unit of each of these factors in this equation for inductance and then volts per amp has units of ohm because ohms law says V equals I R and we can re-arrange that for R to say that it is V divided by I so that’s voltage divided by amps and so volts over amp is ohm and so we have also shown, showing that unit of henry is same as units of ohm second.