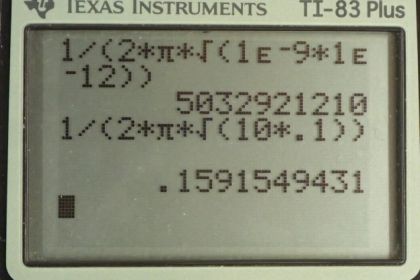
Suppose you have a supply of inductors ranging from 1.00 nH to 10.0 H, and capacitors ranging from 1.00 pF to 0.100 F. What is the range of resonant frequencies that can be achieved from combinations of a single inductor and a single capacitor?

Video Transcript

This is College Physics Answers with Shaun Dychko. Given a collection of inductors ranging from 1 nanohenrys up to 10 henrys and some capacitors from 1 picofarad up to 0.1 farads, what resonant frequency range can we create? So, the resonant frequency is 1 over 2 Pi times square root times of inductance times capacitance. So, the maximum possible resonant frequency we could have is by minimizing this denominator. And so, we want to minimize inductance and capacitance. So, we have 1 over 2 Pi times square root of 1 nanohenry, which is times 10 to the minus 9 henrys and 1 picofarad, which is 1 times 10 to the minus 12 farads. And, this works out to 5.03 gigahertz. The minimum resonant frequency will come by taking the maximum inductance and capacitance so that's 1 over 2 Pi times square root of 10 henrys times 0.1 farads, which is 0.159 hertz.