Question
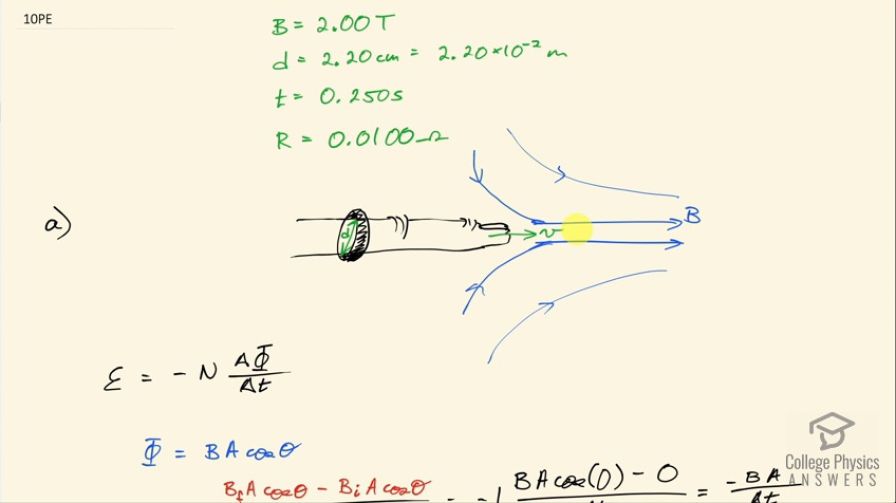
An MRI technician moves his hand from a region of very low magnetic field strength into an MRI scanner’s 2.00 T field with his fingers pointing in the direction of the field. Find the average emf induced in his wedding ring, given its diameter is 2.20 cm and assuming it takes 0.250 s to move it into the field.
Referring to the situation in the previous problem: (a) What current is induced in the ring if its resistance is ? (b) What average power is dissipated? (c) What magnetic field is induced at the center of the ring? (d) What is the direction of the induced magnetic field relative to the MRI’s field?
Final Answer
- The induced field is in the direction opposite to the MRI field in order to oppose the increase in flux.
Solution video
OpenStax College Physics for AP® Courses, Chapter 23, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An MRI technician is wearing a wedding ring and has a finger pointing in the direction of magnetic field inside a MRI machine and they begin with their wedding ring in a region where there is essentially, zero magnetic field and then it goes into the magnetic field and so their finger is going in this direction and this will cause an increase in flux through the wedding ring and that change in flux will result in an induced current and induced magnetic field. Okay! So the field strength is 2.00 tesla, the diameter of the wedding ring is 2.20 centimeters, which is 2.20 times 10 to the minus 2 meters the time it takes for the finger or the wedding ring to go into the field is 0.250 seconds and the resistance in the wedding ring is 0.0100 ohms. So question (a) is what current is induced in the ring? So we have a formula for the induced emf and then we'll combine that with the resistance to figure out the induced current. So emf is the number of turns in a coil times the rate of change in flux— in this case, there's only one turn, it's just one solid band of metal so N is 1— the flux is the magnetic field strength multiplied by the area of the loop times the cosine of the angle between the perpendicular to the plane of the loop and the magnetic field. So the perpendicular to the plane of this wedding ring loop is a line like this and that is parallel to the magnetic field and so this angle Θ is zero and that's what I substitute in over here. So we plug this flux into this change in flux portion of our formula for the emf. So we have the initial magnetic field strength times the area of the ring times cosine Θ minus (did I say initial? I meant final magnetic field) minus the initial magnetic field strength times area times cos Θ and the initial magnetic field strength is zero so this whole term is zero and the final magnetic field strength is just B given to us—2.00 tesla— times the area of the wedding ring that we'll figure out in a second times cos of 0, which is 1 and so this works out to negative BA over Δt. The area of the wedding ring is the area of a circle— π times radius squared— and we are given diameter so we will plug in diameter divided by 2 in place of r and we end up with πd squared over 4 so we substitute that in for A. So the emf then is the magnetic field strength inside the MRI times π times the diameter of the wedding ring squared divided by 4 times the time it takes to insert the wedding ring into the field. Ohm's law says that voltage is current times resistance and we can rearrange this for I by dividing both sides by R and so we have the current then is voltage over resistance and voltage is emf—they are different words for the same thing— and we plug in this Bπd squared over 4Δt in place of V. I am multiplying it by 1 over R just because I don't like to have fractions over fractions so I write it this way instead. So we have 2 tesla times π times 2.20 times 10 to the minus 2 meters squared divided by 4 times 0.250 seconds times 1 over 0.0100 ohms and that is 304 milliamps will be the current induced in the wedding ring. The power dissipated will be this current squared multiplied by its resistance so that's 0.304106 amps squared times 0.0100 ohms which is 9.25 times 10 to the minus 4 watts— a very small amount of power. This would be so small, it wouldn't create any heat that would be noticeable. The magnetic field strength induced inside the wedding ring would be the permeability of free space times the induced current divided by two times the radius of the ring; this is a formula given to us for magnetic field strength inside a current carrying loop. So that's μ naughtI over 2 times half diameter and then these 2's cancel leaving us with μ naughtI over diameter. So that's 4π times 10 to the minus 7 tesla meters per amp times 0.304106 amps over 2.20 times 10 to the minus 2 meters and that's 1.74 times 10 to the minus 5 tesla. Part (d) asks for the direction of the induced field and it's going to be opposite to the MRI field in order to oppose the increase in flux through the loop. So the MRI field is pointing to the right here and so the induced field is pointing to the left.

