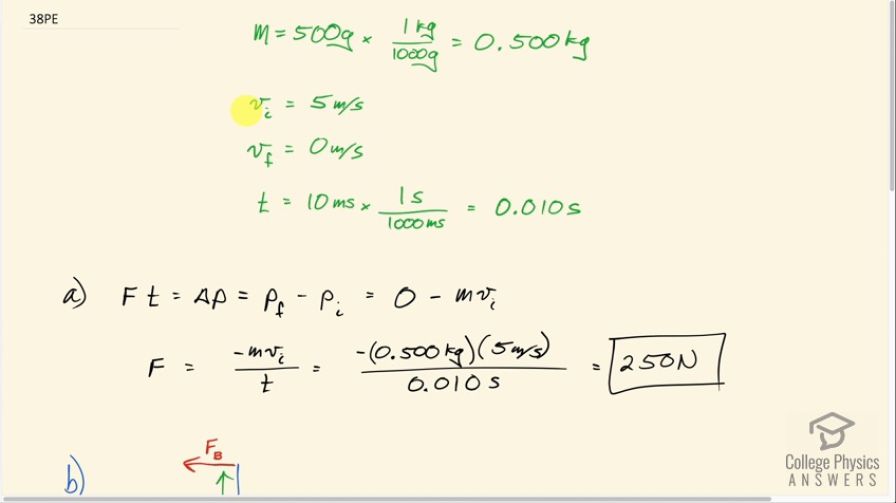
Question
You have just planted a sturdy 2-m-tall palm tree in your front lawn for your mother’s birthday. Your brother kicks a 500 g ball, which hits the top of the tree at a speed of 5 m/s and stays in contact with it for 10 ms. The ball falls to the ground near the base of the tree and the recoil of the tree is minimal. (a) What is the force on the tree? (b) The length of the sturdy section of the root is only 20 cm. Furthermore, the soil around the roots is loose and we can assume that an effective force is applied at the tip of the 20 cm length. What is the effective force exerted by the end of the tip of the root to keep the tree from toppling? Assume the tree will be uprooted rather than bend. (c) What could you have done to ensure that the tree does not uproot easily?
Final Answer
- Compress the soil so that force is exerted all along the length of the root, not only at its tip. One could also install a guy wire to reinforce the tree.
Solution video
OpenStax College Physics for AP® Courses, Chapter 9, Problem 38 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A ball is kicked towards the top of a tree; the ball has a mass of 500 grams which is 0.500 kilograms; it has an initial speed of 5 meters per second and then comes to a stop after hitting a tree— that's what we interpret from when it says the ball falls to the base of the tree— and the time that it's in contact with the top of the tree is 10 milliseconds which we convert into 0.010 seconds. So part (a) is asking us what force does this ball apply on the tree? So impulse is the change in momentum and we can figure out the change in momentum of the ball because that's going to be its mass times its velocity at the two different times after it hits the tree and before it hits the tree and this change in momentum is force multiplied by time— that's a formula we learned from a previous chapter. So we can divide both sides by t and find that the force then is negative mass times initial velocity divided by time. The negative sign indicates the direction but it's not important for this question it's only wanting to know what the magnitude is so let's just put little absolute value signs around all these and then the answer is 250 newtons. So we are substituting in mass of the ball times its initial speed divided by the time it's in contact with the tree; 250 newtons will be applied to the tree. In part (b), we are told that the tree is 2.0 meters high and it has some roots that extend 20.0 centimeters to the side and the ball is applying its force at the top of the tree and the root because the soil is loose is applying a force only at its tip which is this 0.20 meters away from the pivot which we'll take to be here and so our question is, what is going to be the force on the root? So the torque that's counter-clockwise due to the ball has to equal the torque that's clockwise due to this force on the root. This is the case when the root exerts just enough force to keep the tree from falling over and we have the torque due to the ball is the force due to the ball multiplied by this lever arm of the ball, which is 2 meters, and we are taking these forces and lever arms to be perpendicular here. And for the root, we have force of the root multiplied by its distance from the pivot and then we divide both sides by the lever arm of the root and then switch the sides around and we get the force exerted by the root. It's gonna be the torque due to the ball divided by the lever arm of the root so that's 250 newtons times 2 meters divided by 0.20 meters which is 2500 newtons. And part (c) asks what could we do to make it even less likely for the tree to fall over? Well, the soil can be compressed so that there are forces being exerted down all along the length of the root— that would help substantially— and then also a guy wire could be installed so that you have a rope or a metal wire attaching the tree to the ground in which case, it would hold it up. There we go!
