Question
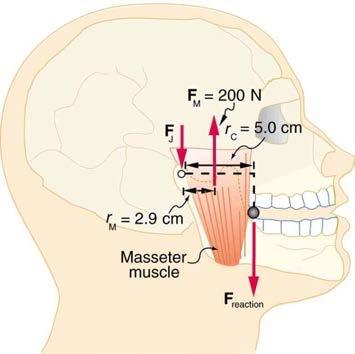
Unlike most of the other muscles in our bodies, the masseter muscle in the jaw, as illustrated in Figure 9.45, is attached relatively far from the joint, enabling large forces to be exerted by the back teeth. (a) Using the information in the figure, calculate the force exerted by the lower teeth on the bullet. (b) Calculate the force on the joint.
Final Answer
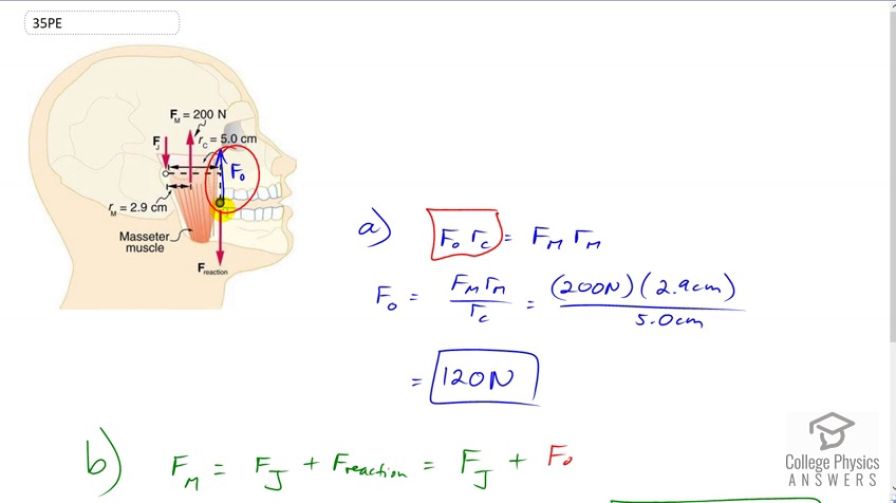
a)
b)
Solution video
OpenStax College Physics for AP® Courses, Chapter 9, Problem 35 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Since this jaw is not going to be moving, the torque exerted by the output force that the bullet will experience from the teeth here -- by the way I have no idea why this guy is eating a bullet, it's a very bad idea. But anyway the torque there is going to equal the torque about the joint of the jaw resulting from the force due to the muscle. So we have the force due to the muscle multiplied by its lever arm r subscript m, and we can divide both sides here by r c to get the output force that the bullet will experience. So that's 200 newtons force exerted by the muscle, multiplied by 2.9 centimeters muscle's lever arm, divided by 5.0 centimeters and that's going to be 120 newtons. Now the force on the joint we'll get by writing down this equation which says that all the up forces equal all the down forces. So the up forces consist of only one force due to the muscle and the down forces are two of them. There is force on the jaw and there is this force downwards on the teeth which is a reaction force to the force that the teeth are applying on the bullet. This reaction force then is the same as F naught. So we will subtract F naught from both sides here and we get the force on the jawbone or jaw joint equals the force on the muscle minus the force on the bullet. So that's 200 newtons minus 116 newtons and that is 84 newtons and this is the force that is downwards.
