Question
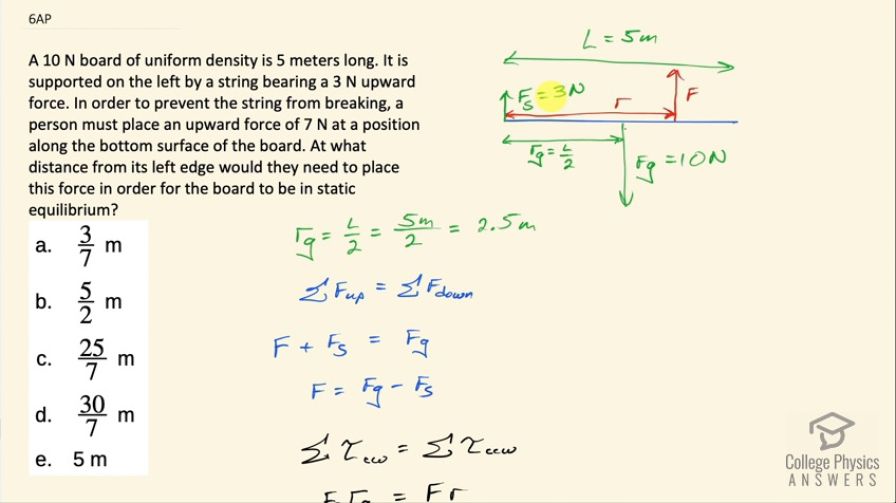
A 10 N board of uniform density is 5 meters long. It is supported on the left by a string bearing a 3 N upward force. In order to prevent the string from breaking, a person must place an upward force of 7 N at a position along the bottom surface of the board. At what distance from its left edge would they need to place this force in order for the board to be in static equilibrium?
  1. 37 m\dfrac{3}{7}\textrm{ m}
  2. 52 m\dfrac{5}{2}\textrm{ m}
  3. 257 m\dfrac{25}{7}\textrm{ m}
  4. 307 m\dfrac{30}{7}\textrm{ m}
  5. 5 m5 \textrm{ m}
Question by OpenStax is licensed under CC BY 4.0
Final Answer

(c)

Solution video

OpenStax College Physics for AP® Courses, Chapter 9, Problem 6 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 9, Problem 6 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Select problem
Next Chevron right
Video Transcript
This is College Physics Answers with Shaun Dychko. A uniform board with a weight of 10 newtons is being held up on the left-end by a string with a force of 3 newtons and the question is where should a new force be applied upwards and at what distance from the left-end such that the board is going to be held level? So because the board is uniform, we know that gravity will act at the geometric center of the board so halfway along its length so its lever-arm r g for gravity is the length of the board L divided by 2 so that's 5 meters divided by 2, which is 2.5 meters. So we know that the net force on the board has to be zero so in other words, the up forces have to equal the total down forces so that's the force that we are trying to find F plus the string force on the left upwards equals the force of gravity downwards and then we can rearrange this to solve for F by subtracting F s from both sides and we get the force then is force of gravity minus the string force. Okay... but we wanna know what this distance r is—that's the main thing— so then let's figure out what that is based on this balancing the torques. So the total clockwise torques have to equal the total counterclockwise torques and there's one torque going clockwise, which is that due to gravity and it's going to be the force of gravity times gravity's lever-arm and the lever-arm and the force are perpendicular so there's no need to write sin Θ because that would just be 1 in this case. And on the right-hand side, we have the force F times its lever-arm r and we can replace F with F g minus F s and then solve for r by dividing both sides by F g minus F s. So the lever-arm then is the force of gravity times the gravity's lever-arm divided by the force of gravity minus the string force. So that's 10 newtons times 2.5 meters divided by 10 minus 3 and that works out to 25 over 7 meters, the answer is (c).