Question
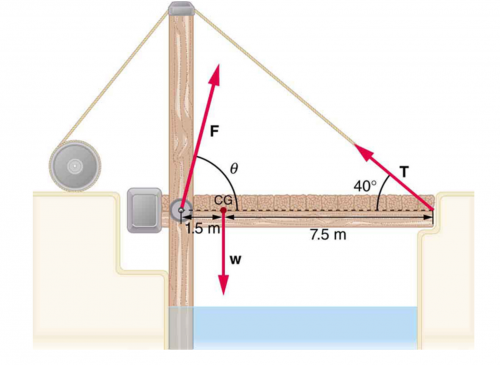
Suppose the weight of the drawbridge in Figure 9.33 is supported entirely by its hinges and the opposite shore, so that its cables are slack. The mass of the bridge is 2500 kg. (a) What fraction of the weight is supported by the opposite shore if the point of support is directly beneath the cable attachments? (b) What is the direction and magnitude of the force the hinges exert on the bridge under these circumstances?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 9, Problem 12 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
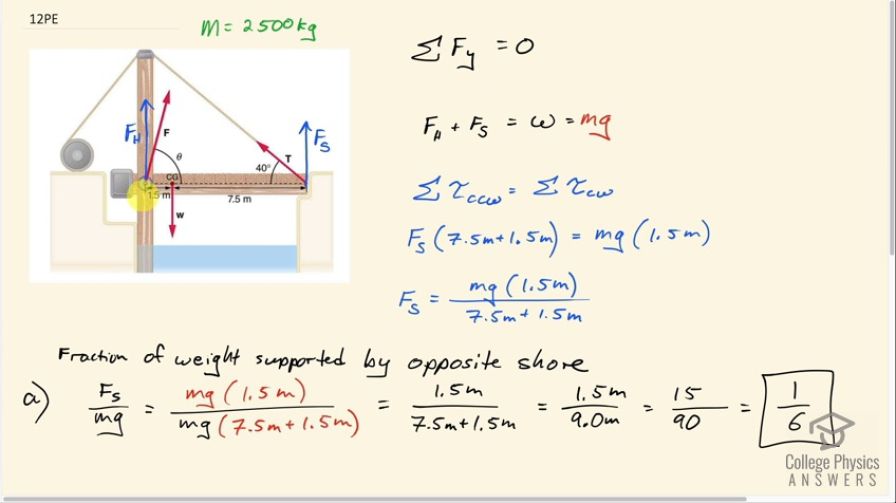
This is College Physics Answers with Shaun Dychko. We are given this picture of a drawbridge and we are told to assume that the cables are slack because the weight is being supported entirely by the hinge and by the opposite shore. So that means there are only two forces upwards: one exerted by the shore straight up and the other exerted by the hinge straight up. And so we'll say that the first condition of equilibrium which is that the vertical forces have to add up to zero that means the hinge force plus the shore force upwards have to equal the weight downwards and the weight downwards is mg. We can also say that the total counter-clockwise torque of which is only 1 that exerted by the shore which is a distance 7.5 plus 1.5 from the hinge— we'll take the pivot to be at the hinge— that's going to equal the clockwise torque which is exerted by the weight which has a force down 1.5 meters from the hinge. So we multiply the lever arm by the forces in each case and then we solve for the force exerted by the shore by dividing both sides by 7.5 plus 1.5 and then we get the shore force is mg times 1.5 over 7.5 plus 1.5. Now the question is not asking us for what the force is though it's asking us for what fraction of the weight is this force and so we have to divide this force by the weight. And so we substitute in this expression for F s and the mg's cancel and we have 1.5 over 9.0 which is the same as 15 over 90 which is the fraction one-sixth. In part (b), we are asked to figure out what is the hinge force... its magnitude and direction and I need to add in that the direction is upwards. Okay! So the hinge force is going to be the weight downwards minus the force exerted by the shore upwards. So that's mg down and this comes from rearranging this here where I subtracted F s from both sides so F H equals mg minus F s. We substitute one-sixth of the weight in place of F s because that's what the fraction is of the force exerted by the shore that's a fraction of one-sixth of the weight and we can factor out the mg and this is mg times five-sixth. So it's 2500 kilograms times 9.80 newtons per kilogram and you know, when you write this as 6 over 6 minus 1 over 6 is 5/6, this works out to 2.0 times 10 to the 4 newtons upwards.
