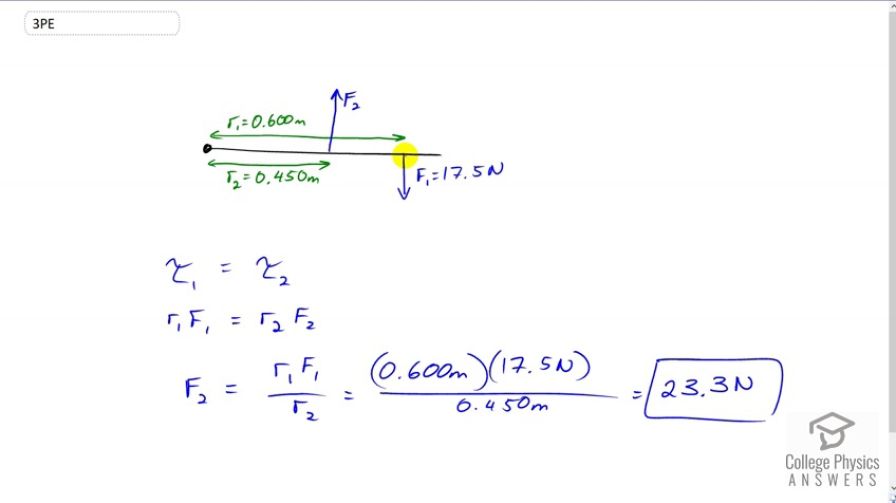
Two children push on opposite sides of a door during play. Both push horizontally and perpendicular to the door. One child pushes with a force of 17.5 N at a distance of 0.600 m from the hinges, and the second child pushes at a distance of 0.450 m. What force must the second child exert to keep the door from moving? Assume friction is negligible.

Video Transcript

This is College Physics Answers with Shaun Dychko. Child one applies a force of 17 and a half newtons at a position 0.6 meters away from the hinge of the door. This force is perpendicular to the door and so we don’t have to worry about any angles here because the angle is 90 degrees just like it need to be for our torque formula. Child two applies a force in the opposite direction, at a position of 0.45 meters away from the hinge, and we want to find out what force should this be such that the torque exerted by child two is the same magnitude as the torque exerted by child one. So we have r1 F1 is the torque from child one and r2 times F2 is the torque from child two, and we’ll divide both sides by r2 to solve for the force from child two. So it’s going to be the torque of child one divided by r2, that’s 0.6 meters times 17 and a half newtons divided by 0.45 meters, that is 23.3 newtons of force from child two to prevent the door from moving.