Question
Suppose you stand with one foot on ceramic flooring and one foot on a wool carpet, making contact over an area of with each foot. Both the ceramic and the carpet are 2.00 cm thick and are on their bottom sides. At what rate must heat transfer occur from each foot to keep the top of the ceramic and carpet at ?
Final Answer
ceramic:
wool:
Solution video
OpenStax College Physics for AP® Courses, Chapter 14, Problem 33 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
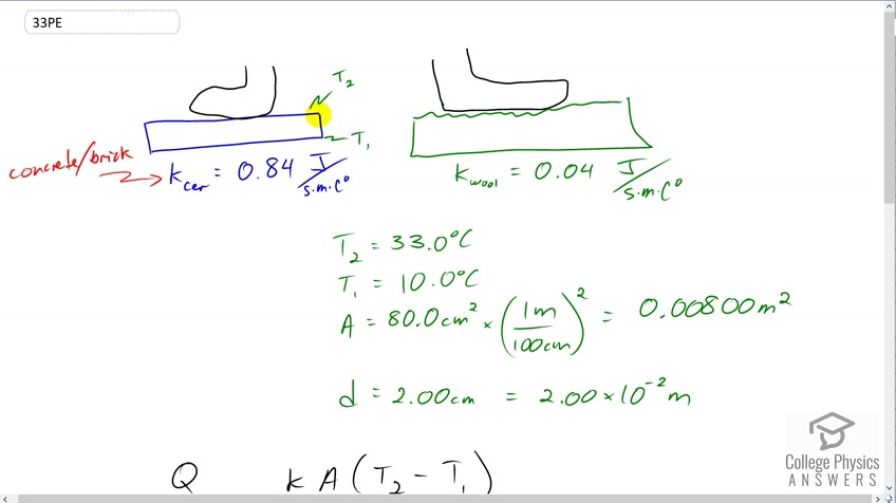
This is College Physics Answers with Shaun Dychko. One foot is standing on a ceramic tile and the other foot is standing on Sobel carpet and the top of the ceramic or wool is meant to be at a temperature of 33 degree Celsius or we are gonna call that T2 because in our formula for thermal rate of thermal conduction, we have T2 representing the body from which the heat leaves and it goes towards body one and so we have heat going from the top so that’s at the higher temperature so that's T2 going towards the bottom of this thickness of ceramic or thickness of wool and the bottom is T1 and that's temperature 10 degree Celsius and so the question is at what rate must heat be be transferred from the foot to the top of the ceramic or wool, in order to maintain this 33 degree Celsius temperature and that'll be the same as the rate with which the top is losing energy to the bottom and so that's why we are calculating this. This is the rate at which energy is transferred from the top of the ceramic to the bottom of the ceramic. And so since the top is losing energy at that rate, it needs to gain that same rate of energy from the foot. Now the foot area is 80 square centimeters which we multiplied by one meter for every 100 centimeters squared to get 0.008 squared meters and the thickness is two centimeters which is two times ten to the minus two meters. Taking care always of unit conversions. In this step we rewrite down the information that we know so we don't have to think about this later. All right. So we have the rate of thermal energy conduction is the thermal conductivity of the material which in the case of ceramic we take to be that of concrete or brick 0.84 Jules per second per meter per Celsius degree and the thermal connectivity of wall is 0.04. OK. So if thermal conductivity multiplied by area times the temperature difference divided by the thickness of a material and so we can substitute for all of these things except for K and to get a formula into which we just plug K for ceramic and then K for wool to get our answers. So if K times 0.008 squared meters times 33 degree Celsius minus 10 degree Celsius divided by two centimeters giving us K multiplied by this 9.2 meters Celsius degrees which are kind of nonsensical units but they are what works out from multiplying and dividing all these things and then when you multiply by K, it will end up being watts. So for ceramic the rate of heat transfer is going to be 0.84 Joules per second per meter per Celsius degree times 9.2 meter Celsius degrees and the meter and Celsius degrees cancel and sure enough the units are joules per second which is watts, 7.7 watts, of energy needs to be given to the top of the ceramic from the foot in order to maintain this rate of heat transfer across the thickness of the ceramic towards the bottom and then for the wool we plug in 0.04 instead of 0.84 and we end up with 0.4 watts and so you can see the wool is a much better insulator due to its lower thermal conductivity and we are seeing that in this rate of heat transfer is much lower than that for ceramic.
