Question
One winter day, the climate control system of a large university classroom building malfunctions. As a result, of excess cold air is brought in each minute. At what rate in kilowatts must heat transfer occur to warm this air by (that is, to bring the air to room temperature)?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 14, Problem 50 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
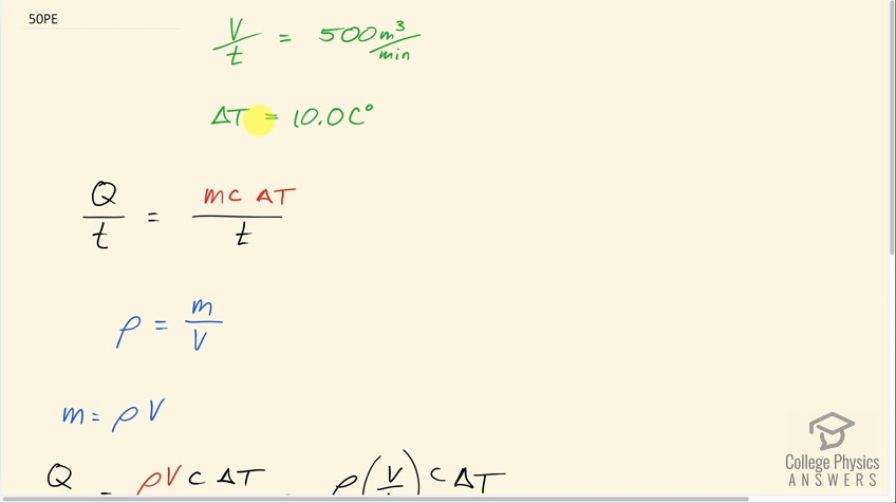
This is College Physics Answers with Shaun Dychko. Every minute, 500 cubic meters of cold air is going into this lecture hall which needs to have its temperature raised by 10.0 Celsius degrees and the question is what rate of energy output is needed from the heating system to do this? So that is a power or it's an energy per time. So the energy per time required is the amount of energy which can be expressed as mass times the specific heat of air times its change in temperature per time. Now we don't know the mass but we do know the volume per time and let's just ignore this time for a second, we'll just talk about volume and we'll make a substitution for mass by knowing the density of air is mass divided by volume which can be rearranged to solve for m and then switch the sides around, we have mass equals density times volume and then we can substitute ρV in place of m. And now we can see that this volume is being divided by time here and I didn't make any real changes here, I just changed the way it looks emphasizing that this V is being divided by the t and this V over t is something that we are given— 500 cubic meters per minute. So we can substitute 500 cubic meters per minute in place of V over t and we multiply by—the density of air— 1.29 kilograms per cubic meters times 721 joules per kilogram per Celsius degrees—specific heat for dry air— times 10.0 Celsius degrees— temperature change— and this will give us an answer in joules per minute if you look at just this part up to here. Now we want our answer in kilowatts though so let's turn this into joules per second first of all to get watts and we get that by multiplying by 1 minute for every 60 seconds and the minute's cancel leaving us with seconds in the denominator and then we multiply by 1 kilowatt for every watt because joules per second is a watt and then that's gonna cancel with these watts and we are left with kilowatts and we could go through and cancel the other units if you just wanna make sure that it works out as well... there we go! So this is 77.5 kilowatts is the power output needed from this heating system to heat 500 cubic meters of air every minute by 10.0 Celsius degrees.
