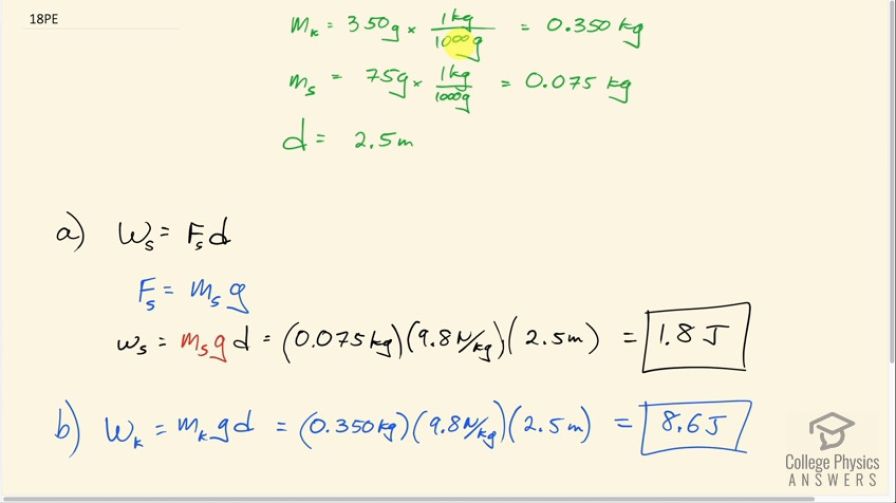
Suppose a 350-g kookaburra (a large kingfisher bird) picks up a 75-g snake and raises it 2.5 m from the ground to a branch. (a) How much work did the bird do on the snake? (b) How much work did it do to raise its own center of mass to the branch?

Video Transcript

This is College Physics Answers with Shaun Dychko. A kookaburra bird likes to eat snakes and the mass of that kookaburra is 350 grams which we convert into kilograms because we need mks units for our formulas— meters, kilograms and seconds. So we multiply by 1 kilogram for every 1000 grams and the kookaburra's mass is 0.35 kilograms. The mass of the snake is 0.75 kilograms and the distance the kookaburra raises the snake onto a branch is 2.50 meters. So the work done on the snake equals the force applied on the snake by the kookaburra multiplied by the distance that it travels. And the force on the snake will be its weight because we assume— even though the question does not explicitly state this— we assume that the force on the snake is equal to the weight of the snake and so in other words, the snake is moving at constant speed upwards, constant velocity. So we substitute that in for the force on the snake and then multiply by the change in height. So that's 0.075 kilograms times 9.8 newtons per kilogram and then we multiply that force by the distance— and these are parallel by the way so they are in the same direction which is good— as I have to be for this calculation for work and we get 1.8 joules. And the work done on the kookaburra is the kookaburra's weight—0.35 kilograms— times 9.80 newtons per kilogram times the height change—of 2.5 meters— which is 8.6 joules.