Question
A car’s bumper is designed to withstand a 4.0-km/h (1.1-m/s) collision with an immovable object without damage to the body of the car. The bumper cushions the shock by absorbing the force over a distance. Calculate the magnitude of the average force on a bumper that collapses 0.200 m while bringing a 900-kg car to rest from an initial speed of 1.1 m/s.
Final Answer
Solution video
OpenStax College Physics, Chapter 7, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
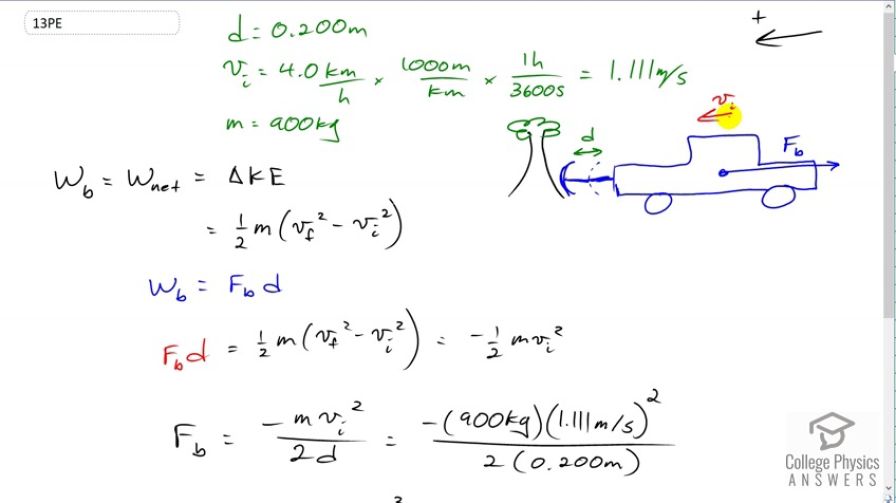
This is College Physics Answers with Shaun Dychko. This car is hitting an immovable object such as a tree and it's going at four kilometers per hour and its initial direction is to the left. We'll say that's positive because that's the way I drew the picture. It comes to a stop within 0.2 meters. So the car travels 0.2 meters and it's only one force on it which is the force due to the bumper. So that makes the force applied by the bumper on the car the net force which means the work done by this force is the net work. We know that net work is the change in kinetic energy. So the change in kinetic energy is one half times mass of the car, times its final speed squared minus its initial speed squared. The work done by the bumper you could also write as the force exerted by the bumper on the car multiplied by the displacement of the car w hich is going to be the amount by which the bumper collapses, d. That's 0.2 meters we're told. So we convert the four kilometers per hour into meters per second by dividing by 3.6 or doing that. That works out to 1.111 meters per second and the mass of the car is 900 kilograms. So we substitute this expression for the work done by the bumper in place of the net work here. So F d or F b, force of the bumper, times d the displacement of the car or the collapsing amount of the bumper is one half mass times final speed squared, minus initial speed squared. But the final speed is zero cause the car comes to a stop. So we can write this as negative one half mass times initial speed squared. Then divide both sides by d and we get the force of the bumper is negative mass, times initial speed squared, divided by two times the displacement. So that's negative 900 kilograms times 1.111 meters per second squared, divided by two times 0.1 meters. This gives negative 2.8 times ten to the three newtons is the force due to the bumper. The question asks us for the magnitude of the force applied by the bumper so we'll take the positive of that to get 2.8 times ten to the three newtons.
Comments
Since the problem doesn't explicitly tell us that the car is moving at 4.0 km/h, shouldn't we be using the speed that we are explicitly told ("an initial speed of 1.1 m/s.") vs the conversion of 4.0 km/h to m/s (1.11...m/s)? If we used the information directly related to the calculation (and not extraneous information about the bumper's design), the calculator answer would be 2,722.5 and the rounded answer would be x
Am I misinterpreting this problem?

