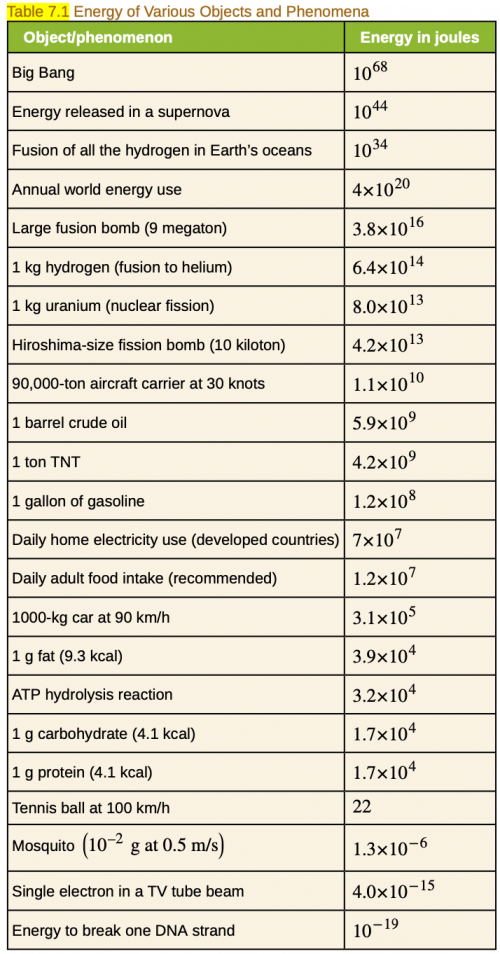
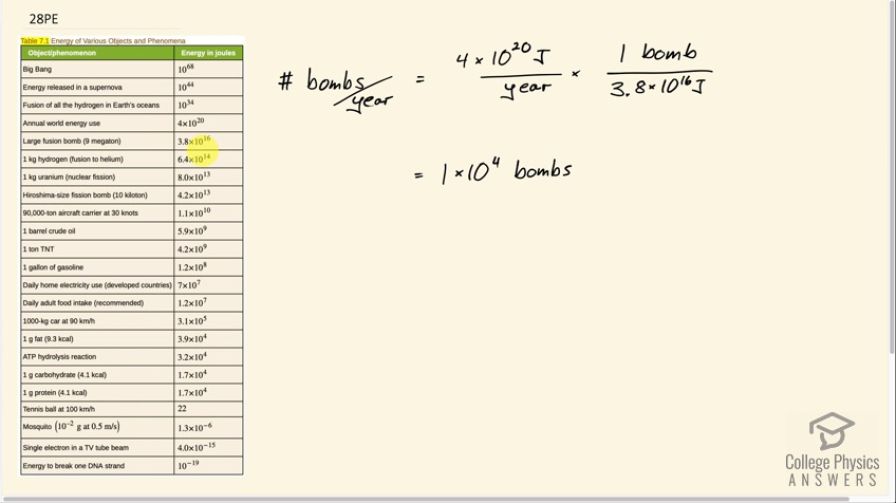
If the energy in fusion bombs were used to supply the energy needs of the world, how many of the 9-megaton variety would be needed for a year’s supply of energy (using data from Table 7.1)? This is not as far-fetched as it may sound—there are thousands of nuclear bombs, and their energy can be trapped in underground explosions and converted to electricity, as natural geothermal energy is.

Video Transcript

This is College Physics Answers with Shaun Dychko. If it was possible to capture the energy from the explosion of a fusion bomb and turn that energy into electricity that the world could use to satisfy its annual energy use, how many bombs would be needed to detonate in order to supply the world's energy for 1 year? So we have 4 times 10 to the 20 joules is the amount of energy the world uses per year approximately and we'll multiply that by 1 bomb for every 3.8 times 10 to the 16 joules making the assumption that all of the energy from the explosion could be converted into electricity with perfect efficiency and this works out to 1 times 10 to the 4 bombs so that would mean 10,000 bombs. And this is not quite as far fetched as it seems because these bombs could be put deep underground and exploded there which would create heat that could then be captured in the same way that geothermal heat is captured and it would take 10,000 bombs to provide enough heat energy to convert into electricity for satisfying the world's annual energy use.