Question
(a) How much gravitational potential energy (relative to the ground on which it is built) is stored in the Great Pyramid of Cheops, given that its mass is about and its center of mass is 36.5 m above the surrounding ground? (b) How does this energy compare with the daily food intake of a person?
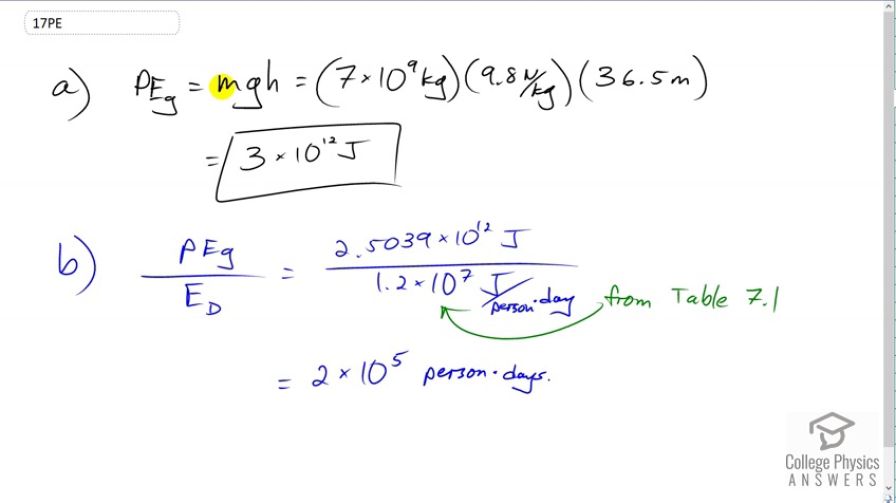
Final Answer
a)
b)
Solution video
OpenStax College Physics, Chapter 7, Problem 17 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The total gravitational potential energy stored in the stones of the Great Pyramid of Cheops is equal to the total mass of all the stones times the gravitational field strength, times the height of the center of mass. So that's seven times ten to the nine kilograms times 9.8 newtons per kilogram, times 36.5 meters, which is about three times ten to the twelve joules. Now, if we compare that to the average daily intake for an adult of energy, we can get a minimum value for the -- we can make an estimate of how many people would have been needed to build this pyramid given a period of time that we choose. I'll explain that in a second. So, first of all just to take this ratio, we have 2.5039 times ten to the twelve joules where I've used the un-rounded answer from part A and dividing it by 1.2 times ten to the seven joules recommended per person per day, that's from Table 7.1, and this gives two times ten to the five person days. So that means if you had one thousand ancient Egyptians working then they would need to take a theoretical minimum of 200 days to impart this much energy to all the stones of the pyramid. So this would make two times ten to the five person days. Now, of course this is unrealistic because this calculation assumes perfect efficiency and that's not going to happen. But it at least sets a theoretical minimum that if there were a thousand people working it would take longer than two hundred days for sure. If there were two thousand people working then it would take longer than one hundred days and so on.
Comments
When I examine the significant figures in your video versus in the answer key, I see 3x10^12 in the video and 3.x10^12 in the answer key. Which one is correct, and why?
Notice that the given 7x10^9 doesn't have a decimal point in it. Shouldn't the final answer, therefore, be without a decimal point?
Hello PlaywtPhysics,
Thank you for the question. There is no difference in the number of significant figures between vs . Both have only 1 significant figure. The difference is merely one of personal preference, with the vast majority of people choosing not to write the trailing decimal.
All the best,
Shaun


