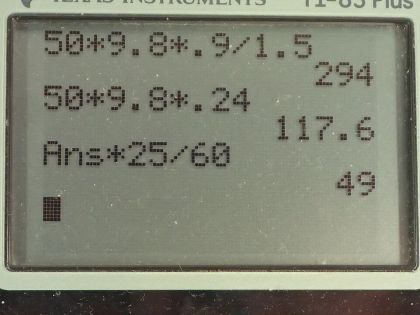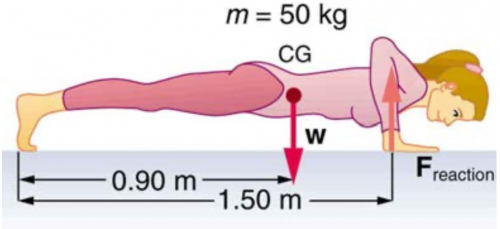
- . This answer is open to discussion, and depends on your assumptions about the biomechanics involved during the recovery phase of the push-up. If the descent is seen as a resting phase with a negligible effort, then would be the best answer.
Solution video
OpenStax College Physics, Chapter 7, Problem 60 (Problems & Exercises)
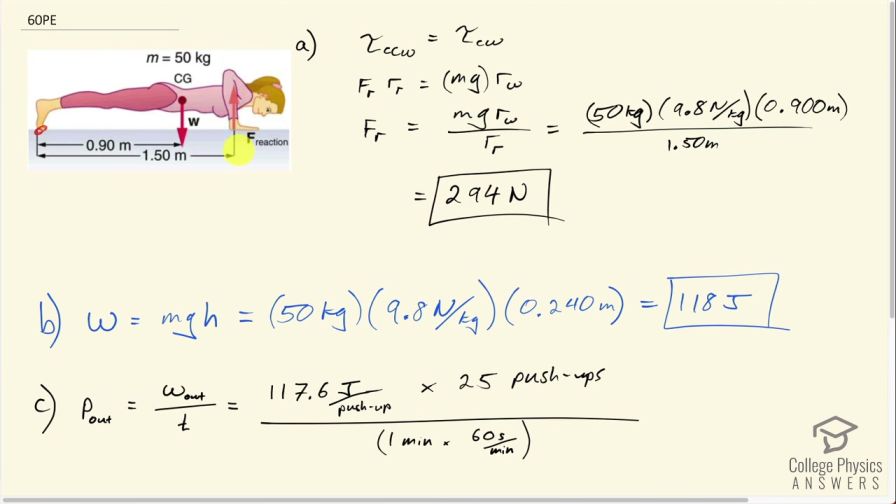
Calculator Screenshots
Comments
This answer is wrong for part A. The text requires you to take CG divided by 1.50m and then multiply that by the total N.
Hello phlips79, thank you very much for noticing this. I've put the following note in the final answer "In part (a) the video makes the mistake of making the force she applies with her hands equal to the weight, but this ignores the fact that her feet are also experiencing a normal force from the ground, so the force applied by her hands will be less than her weight. Using torque is the correct approach to solving part (a), rather than saying net force has to be zero."
This answer is also wrong for part B. The text requires you to take m*g*h.
The answer is wrong for part C. The text does NOT assume work is done both upward and downward, it only assumes work is done upward. So the answer is ((117.6J)*(25 pushups))/60 = 49W
Hi phlips79, another excellent comment, thank you. Here is the note I've added to the final answer: "This answer is open to discussion, and depends on your assumptions about the biomechanics involved during the recovery phase of the push-up. If the descent is truly at constant speed, then the athlete will need to exert the same force she did during the rising phase of the push-up, in which case 98.0 W is the correct answer. However, if the descent is seen as a resting phase with a negligible effort put into changing direction at the bottom of the pushup, then 49.0 W would be the correct answer."
In the textbook, the only guidance I see is "Useful work requires a force exerted through a distance on the outside world" which occurs on both the upward and downward phases of the push-up, but I concede that it's open to interpretation. I think the important thing, as is the case with most real-world problems which have non-idealized complicating factors, is that you're able to make a logical defence for your approach and assumptions.
Thanks again for your feedback,
Shaun
That is correct
Just make another video. Simple as that
Hi Joe, you're totally right. I'm in the process of upgrading the website technology at the moment, and intend to fix errata after that.
All the best,
Shaun
This video was updated on Dec. 20th, 2023.