Question
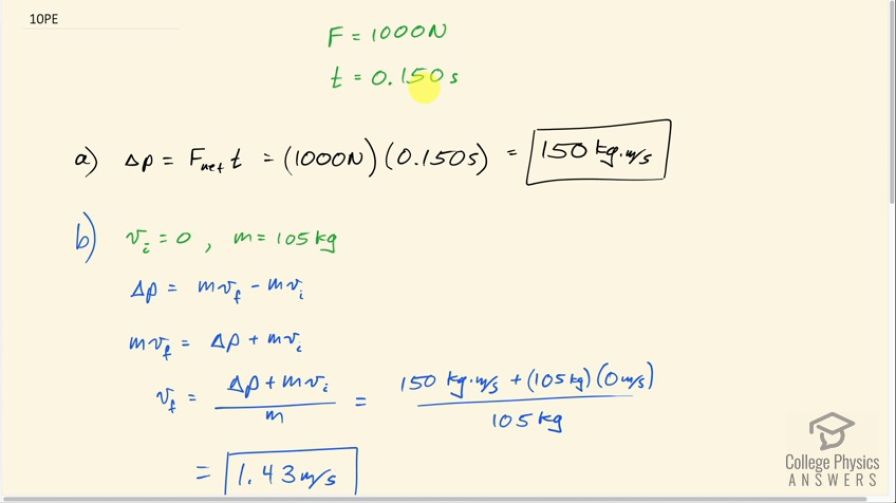
A professional boxer hits his opponent with a 1000-N horizontal blow that lasts for 0.150 s. (a) Calculate the impulse imparted by this blow. (b) What is the opponent’s final velocity, if his mass is 105 kg and he is motionless in midair when struck near his center of mass? (c) Calculate the recoil velocity of the opponent’s 10.0-kg head if hit in this manner, assuming the head does not initially transfer significant momentum to the boxer’s body. (d) Discuss the implications of your answers for parts (b) and (c).
Final Answer
- The velocity when impacting only the head is significant and would result in concussion injury when imparted in such a short time. To achieve a knockout, boxers should strategically aim for the head.
Solution video
OpenStax College Physics, Chapter 8, Problem 10 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A boxer hits an opponent with a force of 1000 newtons and the hit lasts for 0.150 seconds. So part (a) asks us what is the impulse given to the opponent? So that's gonna equal the net force applied on them multiplied by time and this force of the hit is going to be the net force because there's only going to be one horizontal force involved here. So we have a 1000 newtons times 0.150 seconds which is 150 kilogram meters per second or you could write these units as newton seconds if you prefer. Part (b) says suppose the opponent is in the air, I guess they have jumped, and they have an initial velocity of zero and a mass of 105 kilograms and this hit strikes them near their center of mass so it's going to move their whole body, what will their final speed be? So impulse is the final momentum minus the initial momentum and we can solve for mv f by adding mv i to both sides and then switching the sides around and then solve for v f by dividing both sides by m. So the final speed will be the impulse plus mass times initial velocity divided by mass. So it's a 150 kilogram meters per second— impulse that we calculated in part (a)— plus 0 because the initial velocity is zero divided by 105 kilograms and that is 1.43 meters per second. And then part (c) says what would the final velocity of their head be if they were struck in the head instead of in the center of mass of their body? So it's the same impulse because it's the same force applied for the same amount of time but now we are adding 10.0 kilograms multiplied by 0—initial velocity— divided by 10.0 kilograms here and we get a new final velocity of 15.0 meters per second. And that is a significant speed; if we convert it into kilometers per hour, we see that it's 54.0 kilometers an hour and that velocity is significant especially when it's imparted in this short duration of time of only 0.150 seconds. And that would probably result in a concussion injury but you know, unfortunately, that's the actual point in boxing is to cause concussion's because that's what it means to blackout and have a knockout is when somebody's had enough concussion injury that they go unconscious. So I guess the lesson here for a boxer that wants to be strategic is that to achieve a knockout, they should aim for the head because the head will go much faster when the impulse is imparted only to the head versus when it's imparted to the whole body.
